Question
Two non-intersecting circles C1 , containing points M and S , and C2 , containing points N and R, have centres P and Q where PQ \( = 50\) . The line segments [MN] and [SR] are common tangents to the circles. The size of the reflex angle MPS is \( \alpha\), the size of the obtuse angle NQR is \( \beta\) , and the size of the angle MPQ is \( \theta\) . The arc length MS is \({l_1}\) and the arc length NR is \({l_2}\) . This information is represented in the diagram below.
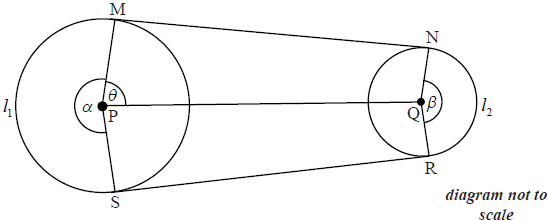
The radius of C1 is \(x\) , where \(x \geqslant 10\) and the radius of C2 is \(10\).
(a) Explain why \(x < 40\) .
(b) Show that cosθ = x −10 50.
(c) (i) Find an expression for MN in terms of \(x\) .
(ii) Find the value of \(x\) that maximises MN.
(d) Find an expression in terms of \(x\) for
(i) \( \alpha\) ;
(ii) \( \beta\) .
(e) The length of the perimeter is given by \({l_1} + {l_2} + {\text{MN}} + {\text{SR}}\).
(i) Find an expression, \(b (x)\) , for the length of the perimeter in terms of \(x\) .
(ii) Find the maximum value of the length of the perimeter.
(iii) Find the value of \(x\) that gives a perimeter of length \(200\).
▶️Answer/Explanation
Markscheme
(a) PQ \( = 50\) and non-intersecting R1
[1 mark]
(b) a construction QT (where T is on the radius MP), parallel to MN, so that \({\text{Q}}\hat {\text{T}}{\text{M}} = 90^\circ \) (angle between tangent and radius \( = 90^\circ \) ) M1
lengths \(50\), \(x – 10\) and angle \( \theta\) marked on a diagram, or equivalent R1
Note: Other construction lines are possible.
[2 marks]
(c) (i) MN \( = \sqrt {{{50}^2} – {{\left( {x – 10} \right)}^2}} \) A1
(ii) maximum for MN occurs when \(x = 10\) A1
[2 marks]
(d) (i) \(\alpha = 2\pi – 2\theta \) M1
\( = 2\pi – 2\arccos \left( {\frac{{x – 10}}{{50}}} \right)\) A1
(ii) \(\beta = 2\pi – \alpha \) ( \( = 2\theta \) ) A1
\( = 2\left( {{{\cos }^{ – 1}}\left( {\frac{{x – 10}}{{50}}} \right)} \right)\) A1
[4 marks]
(e) (i) \(b(x) = x\alpha + 10\beta + 2\sqrt {{{50}^2} – {{\left( {x – 10} \right)}^2}} \) A1A1A1
\( = x\left( {2\pi – 2\left( {{{\cos }^{ – 1}}\left( {\frac{{x – 10}}{{50}}} \right)} \right)} \right) + 20\left( {\left( {{{\cos }^{ – 1}}\left( {\frac{{x – 10}}{{50}}} \right)} \right)} \right) + 2\sqrt {{{50}^2} – {{\left( {x – 10} \right)}^2}} \) M1A1
(ii) maximum value of perimeter \( = 276\) A2
(iii) perimeter of \(200\) cm \(b(x) = 200\) (M1)
when \(x = 21.2\) A1
[9 marks]
Total [18 marks]
Examiners report
This is not an inherently difficult question, but candidates either made heavy weather of it or avoided it almost entirely. The key to answering the question is in obtaining the displayed answer to part (b), for which a construction line parallel to MN through Q is required. Diagrams seen by examiners on some scripts tend to suggest that the perpendicularity property of a tangent to a circle and the associated radius is not as firmly known as they had expected. Some candidates mixed radians and degrees in their expressions.
Question
The diagram below shows two concentric circles with centre O and radii 2 cm and 4 cm.
The points P and Q lie on the larger circle and \({\rm{P}}\hat {\text{O}}{\text{Q}} = x\) , where \(0 < x < \frac{\pi }{2}\) .
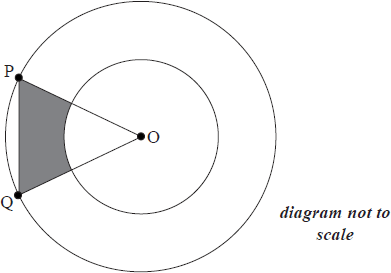
(a) Show that the area of the shaded region is \(8\sin x – 2x\) .
(b) Find the maximum area of the shaded region.
▶️Answer/Explanation
Markscheme
(a) shaded area area of triangle area of sector, i.e. (M1)
\(\left( {\frac{1}{2} \times {4^2}\sin x} \right) – \left( {\frac{1}{2}{2^2}x} \right) = 8\sin x – 2x\) A1A1AG
(b) EITHER
any method from GDC gaining \(x \approx 1.32\) (M1)(A1)
maximum value for given domain is \(5.11\) A2
OR
\(\frac{{{\text{d}}A}}{{{\text{d}}x}} = 8\cos x – 2\) A1
set \(\frac{{{\text{d}}A}}{{{\text{d}}x}} = 0\), hence \(8\cos x – 2 = 0\) M1
\(\cos x = \frac{1}{4} \Rightarrow x \approx 1.32\) A1
hence \({A_{\max }} = 5.11\) A1
[7 marks]
Examiners report
Generally a well answered question.
Question
The interior of a circle of radius 2 cm is divided into an infinite number of sectors. The areas of these sectors form a geometric sequence with common ratio k. The angle of the first sector is \(\theta \) radians.
(a) Show that \(\theta = 2\pi (1 – k)\).
(b) The perimeter of the third sector is half the perimeter of the first sector.
Find the value of k and of \(\theta \).
▶️Answer/Explanation
Markscheme
(a) the area of the first sector is \(\frac{1}{2}{2^2}\theta \) (A1)
the sequence of areas is \(2\theta ,{\text{ }}2k\theta ,{\text{ }}2{k^2}\theta \ldots \) (A1)
the sum of these areas is \(2\theta (1 + k + {k^2} + \ldots )\) (M1)
\( = \frac{{2\theta }}{{1 – k}} = 4\pi \) M1A1
hence \(\theta = 2\pi (1 – k)\) AG
Note: Accept solutions where candidates deal with angles instead of area.
[5 marks]
(b) the perimeter of the first sector is \(4 + 2\theta \) (A1)
the perimeter of the third sector is \(4 + 2{k^2}\theta \) (A1)
the given condition is \(4 + 2{k^2}\theta = 2 + \theta \) M1
which simplifies to \(2 = \theta (1 – 2{k^2})\) A1
eliminating \(\theta \), obtain cubic in k: \(\pi (1 – k)(1 – 2{k^2}) – 1 = 0\) A1
or equivalent
solve for k = 0.456 and then \(\theta = 3.42\) A1A1
[7 marks]
Total [12 marks]
Question
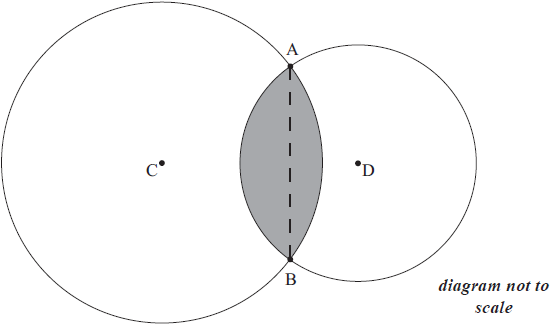
The radius of the circle with centre C is 7 cm and the radius of the circle with centre D is 5 cm. If the length of the chord [AB] is 9 cm, find the area of the shaded region enclosed by the two arcs AB.
▶️Answer/Explanation
Markscheme

\(\alpha = 2\arcsin \left( {\frac{{4.5}}{7}} \right)\) (\( \Rightarrow \alpha = 1.396… = 80.010^\circ …\)) M1(A1)
\(\beta = 2\arcsin \left( {\frac{{4.5}}{5}} \right)\) (\( \Rightarrow \beta = 2.239… = 128.31^\circ …\)) (A1)
Note: Allow use of cosine rule.
area \(P = \frac{1}{2} \times {7^2} \times \left( {\alpha – \sin \alpha } \right) = 10.08…\) M1(A1)
area \(Q = \frac{1}{2} \times {5^2} \times \left( {\beta – \sin \beta } \right) = 18.18…\) (A1)
Note: The M1 is for an attempt at area of sector minus area of triangle.
Note: The use of degrees correctly converted is acceptable.
area = 28.3 (cm2) A1
[7 marks]
Examiners report
Whilst most candidates were able to make the correct construction to solve the problem some candidates seemed unable to find the area of a segment. In a number of cases candidates used degrees in a formula that required radians. There were a number of candidates who followed a completely correct method but due to premature approximation were unable to obtain a correct solution.
Question
The points P and Q lie on a circle, with centre O and radius 8 cm, such that \({\rm{P\hat OQ}} = 59^\circ \) .
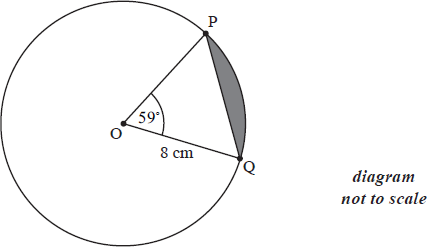
Find the area of the shaded segment of the circle contained between the arc PQ and the chord [PQ].
▶️Answer/Explanation
Markscheme
area of triangle \({\text{POQ}} = \frac{1}{2}{8^2}\sin 59^\circ \) M1
= 27.43 (A1)
area of sector \( = \pi {8^2}\frac{{59}}{{360}}\) M1
= 32.95 (A1)
area between arc and chord \( = 32.95 – 27.43\)
\( = 5.52{\text{ (c}}{{\text{m}}^2})\) A1
[5 marks]
Examiners report
This was an easy starter question, with most candidates gaining full marks. Others lost marks through premature rounding or the incorrect use of radian measure.
