Question
a.Using the definition of a derivative as \(f'(x) = \mathop {\lim }\limits_{h \to 0} \left( {\frac{{f(x + h) – f(x)}}{h}} \right)\) , show that the derivative of \(\frac{1}{{2x + 1}}{\text{ is }}\frac{{ – 2}}{{{{(2x + 1)}^2}}}\).[4]
b.Prove by induction that the \({n^{{\text{th}}}}\) derivative of \({(2x + 1)^{ – 1}}\) is \({( – 1)^n}\frac{{{2^n}n!}}{{{{(2x + 1)}^{n + 1}}}}\).[9]
▶️Answer/Explanation
Markscheme
let \(f(x) = \frac{1}{{2x + 1}}\) and using the result \(f'(x) = \mathop {\lim }\limits_{h \to 0} \left( {\frac{{f(x + h) – f(x)}}{h}} \right)\)
\(f'(x) = \mathop {\lim }\limits_{h \to 0} \left( {\frac{{\frac{1}{{2(x + h) + 1}} – \frac{1}{{2x + 1}}}}{h}} \right)\) M1A1
\( \Rightarrow f'(x) = \mathop {\lim }\limits_{h \to 0} \left( {\frac{{[2x + 1] – [2(x + h) + 1]}}{{h[2(x + h) + 1][2x + 1]}}} \right)\) A1
\( \Rightarrow f'(x) = \mathop {\lim }\limits_{h \to 0} \left( {\frac{{ – 2}}{{[2(x + h) + 1][2x + 1]}}} \right)\) A1
\( \Rightarrow f'(x) = \frac{{ – 2}}{{{{(2x + 1)}^2}}}\) AG
[4 marks]
let \(y = \frac{1}{{2x + 1}}\)
we want to prove that \(\frac{{{{\text{d}}^n}y}}{{{\text{d}}{x^n}}} = {( – 1)^n}\frac{{{2^n}n!}}{{{{(2x + 1)}^{n + 1}}}}\)
let \(n = 1 \Rightarrow \frac{{{\text{d}}y}}{{{\text{d}}x}} = {( – 1)^1}\frac{{{2^1}1!}}{{{{(2x + 1)}^{1 + 1}}}}\) M1
\( \Rightarrow \frac{{{\text{d}}y}}{{{\text{d}}x}} = \frac{{ – 2}}{{{{(2x + 1)}^2}}}\) which is the same result as part (a)
hence the result is true for \(n = 1\) R1
assume the result is true for \(n = k{\text{ : }}\frac{{{{\text{d}}^k}y}}{{{\text{d}}{x^k}}} = {( – 1)^k}\frac{{{2^k}k!}}{{{{(2x + 1)}^{k + 1}}}}\) M1
\(\frac{{{{\text{d}}^{k + 1}}y}}{{{\text{d}}{x^{k + 1}}}} = \frac{{\text{d}}}{{{\text{d}}x}}\left[ {{{( – 1)}^k}\frac{{{2^k}k!}}{{{{(2x + 1)}^{k + 1}}}}} \right]\) M1
\( \Rightarrow \frac{{{{\text{d}}^{k + 1}}y}}{{{\text{d}}{x^{k + 1}}}} = \frac{{\text{d}}}{{{\text{d}}x}}\left[ {{{( – 1)}^k}{2^k}k!{{(2x + 1)}^{ – k – 1}}} \right]\) (A1)
\( \Rightarrow \frac{{{{\text{d}}^{k + 1}}y}}{{{\text{d}}{x^{k + 1}}}} = {( – 1)^k}{2^k}k!( – k – 1){(2x + 1)^{ – k – 2}} \times 2\) A1
\( \Rightarrow \frac{{{{\text{d}}^{k + 1}}y}}{{{\text{d}}{x^{k + 1}}}} = {( – 1)^{k + 1}}{2^{k + 1}}(k + 1)!{(2x + 1)^{ – k – 2}}\) (A1)
\( \Rightarrow \frac{{{{\text{d}}^{k + 1}}y}}{{{\text{d}}{x^{k + 1}}}} = {( – 1)^{k + 1}}\frac{{{2^{k + 1}}(k + 1)!}}{{{{(2x + 1)}^{k + 2}}}}\) A1
hence if the result is true for \(n = k\) , it is true for \(n = k + 1\)
since the result is true for \(n = 1\) , the result is proved by mathematical induction R1
Note: Only award final R1 if all the M marks have been gained.
[9 marks]
Examiners report
Even though the definition of the derivative was given in the question, solutions to (a) were often disappointing with algebraic errors fairly common, usually due to brackets being omitted or manipulated incorrectly. Solutions to the proof by induction in (b) were often poor. Many candidates fail to understand that they have to assume that the result is true for \(n = k\) and then show that this leads to it being true for \(n = k + 1\). Many candidates just write ‘Let \(n = k\)’ which is of course meaningless. The conclusion is often of the form ‘True for \(n = 1,{\text{ }}n = k{\text{ and }}n = k + 1\) therefore true by induction’. Credit is only given for a conclusion which includes a statement such as ‘True for \(n = k \Rightarrow \) true for \(n = k + 1\)’.
Even though the definition of the derivative was given in the question, solutions to (a) were often disappointing with algebraic errors fairly common, usually due to brackets being omitted or manipulated incorrectly. Solutions to the proof by induction in (b) were often poor. Many candidates fail to understand that they have to assume that the result is true for \(n = k\) and then show that this leads to it being true for \(n = k + 1\). Many candidates just write ‘Let \(n = k\)’ which is of course meaningless. The conclusion is often of the form ‘True for \(n = 1,{\text{ }}n = k{\text{ and }}n = k + 1\) therefore true by induction’. Credit is only given for a conclusion which includes a statement such as ‘True for \(n = k \Rightarrow \) true for \(n = k + 1\)’.
Question
Find the \(x\)-coordinates of all the points on the curve \(y = 2{x^4} + 6{x^3} + \frac{7}{2}{x^2} – 5x + \frac{3}{2}\) at which
the tangent to the curve is parallel to the tangent at \(( – 1,{\text{ }}6)\).
▶️Answer/Explanation
Markscheme
\(\frac{{{\text{d}}y}}{{{\text{d}}x}} = 8{x^3} + 18{x^2} + 7x – 5\) A1
when \(x = – 1,{\text{ }}\frac{{{\text{d}}y}}{{{\text{d}}x}} = – 2\) A1
\(8{x^3} + 18{x^2} + 7x – 5 = – 2\) M1
\(8{x^3} + 18{x^2} + 7x – 3 = 0\)
\((x + 1)\) is a factor A1
\(8{x^3} + 18{x^2} + 7x – 3 = (x + 1)(8{x^2} + 10x – 3)\) (M1)
Note: M1 is for attempting to find the quadratic factor.
\((x + 1)(4x – 1)(2x + 3) = 0\)
\((x = – 1),{\text{ }}x = 0.25,{\text{ }}x = – 1.5\) (M1)A1
Note: M1 is for an attempt to solve their quadratic factor.
[7 marks]
Examiners report
The first half of the question was accessible to all the candidates. Some though saw the word ‘tangent’ and lost time calculating the equation of this. It was a pity that so many failed to spot that \(x + 1\) was a factor of the cubic and so did not make much progress with the final part of this question.
Question
The function \(f\) is defined as \(f(x) = a{x^2} + bx + c\) where \(a,{\text{ }}b,{\text{ }}c \in \mathbb{R}\).
Hayley conjectures that \(\frac{{f({x_2}) – f({x_1})}}{{{x_2} – {x_1}}} = \frac{{f'({x_2}) + f'({x_1})}}{2},{\text{ }}x1 \ne x2\).
Show that Hayley’s conjecture is correct.
▶️Answer/Explanation
Markscheme
\(\frac{{f({x_2}) – f({x_1})}}{{{x_2} – {x_1}}} = \frac{{ax_2^2 + b{x_2} + c – (ax_1^2 + b{x_1} + c)}}{{{x_2} – {x_1}}}\) (M1)
\( = \frac{{a(x_2^2 – x_1^2) + b({x_2} – {x_1})}}{{{x_2} – {x_1}}}\) A1
\( = \frac{{a({x_2} – {x_1})({x_2} + {x_1}) + b({x_2} – {x_1})}}{{{x_2} – {x_1}}}\) (A1)
\( = a({x_2} + {x_1}) + b\,\,\,\,\,({x_1} \ne {x_2})\) A1
\(\frac{{f'({x_2}) + f'({x_1})}}{2} = \frac{{(2a{x_2} + b) + (2a{x_1} + b)}}{2}\) M1
\( = \frac{{2a({x_2} + {x_1}) + 2b}}{2}\)
\( = a({x_2} + {x_1}) + b\) A1
so Hayley’s conjecture is correct AG
[6 marks]
Examiners report
This was generally answered very well. A small minority attempted to ‘prove’ the result by substituting specific values into the identity and thus gained little or no credit. Some started by assuming the result to be correct, then manipulated both sides until they derived an obvious identity. Reluctantly, they gained credit for this, though such an approach should be discouraged.
Question
The function \(f\) is defined as \(f(x) = a{x^2} + bx + c\) where \(a,{\text{ }}b,{\text{ }}c \in \mathbb{R}\).
Hayley conjectures that \(\frac{{f({x_2}) – f({x_1})}}{{{x_2} – {x_1}}} = \frac{{f'({x_2}) + f'({x_1})}}{2},{\text{ }}x1 \ne x2\).
Show that Hayley’s conjecture is correct.
▶️Answer/Explanation
Markscheme
\(\frac{{f({x_2}) – f({x_1})}}{{{x_2} – {x_1}}} = \frac{{ax_2^2 + b{x_2} + c – (ax_1^2 + b{x_1} + c)}}{{{x_2} – {x_1}}}\) (M1)
\( = \frac{{a(x_2^2 – x_1^2) + b({x_2} – {x_1})}}{{{x_2} – {x_1}}}\) A1
\( = \frac{{a({x_2} – {x_1})({x_2} + {x_1}) + b({x_2} – {x_1})}}{{{x_2} – {x_1}}}\) (A1)
\( = a({x_2} + {x_1}) + b\,\,\,\,\,({x_1} \ne {x_2})\) A1
\(\frac{{f'({x_2}) + f'({x_1})}}{2} = \frac{{(2a{x_2} + b) + (2a{x_1} + b)}}{2}\) M1
\( = \frac{{2a({x_2} + {x_1}) + 2b}}{2}\)
\( = a({x_2} + {x_1}) + b\) A1
so Hayley’s conjecture is correct AG
[6 marks]
Examiners report
This was generally answered very well. A small minority attempted to ‘prove’ the result by substituting specific values into the identity and thus gained little or no credit. Some started by assuming the result to be correct, then manipulated both sides until they derived an obvious identity. Reluctantly, they gained credit for this, though such an approach should be discouraged.
Question
A curve has equation \(3x – 2{y^2}{{\text{e}}^{x – 1}} = 2\).
Find an expression for \(\frac{{{\text{d}}y}}{{{\text{d}}x}}\) in terms of \(x\) and \(y\).
Find the equations of the tangents to this curve at the points where the curve intersects the line \(x = 1\).
▶️Answer/Explanation
Markscheme
attempt to differentiate implicitly M1
\(3 – \left( {4y\frac{{{\text{d}}y}}{{{\text{d}}x}} + 2{y^2}} \right){{\text{e}}^{x – 1}} = 0\) A1A1A1
Note: Award A1 for correctly differentiating each term.
\(\frac{{{\text{d}}y}}{{{\text{d}}x}} = \frac{{3 \bullet {{\text{e}}^{1 – x}} – 2{y^2}}}{{4y}}\) A1
Note: This final answer may be expressed in a number of different ways.
[5 marks]
\(3 – 2{y^2} = 2 \Rightarrow {y^2} = \frac{1}{2} \Rightarrow y = \pm \sqrt {\frac{1}{2}} \) A1
\(\frac{{{\text{d}}y}}{{{\text{d}}x}} = \frac{{3 – 2 \bullet \frac{1}{2}}}{{ \pm 4\sqrt {\frac{1}{2}} }} = \pm \frac{{\sqrt 2 }}{2}\) M1
at \(\left( {1,{\text{ }}\sqrt {\frac{1}{2}} } \right)\) the tangent is \(y – \sqrt {\frac{1}{2}} = \frac{{\sqrt 2 }}{2}(x – 1)\) and A1
at \(\left( {1,{\text{ }} – \sqrt {\frac{1}{2}} } \right)\) the tangent is \(y + \sqrt {\frac{1}{2}} = – \frac{{\sqrt 2 }}{2}(x – 1)\) A1
Note: These equations simplify to \(y = \pm \frac{{\sqrt 2 }}{2}x\).
Note: Award A0M1A1A0 if just the positive value of \(y\) is considered and just one tangent is found.
[4 marks]
Examiners report
[N/A]
[N/A]
Question
Let \(y = {{\text{e}}^x}\sin x\).
Consider the function \(f\) defined by \(f(x) = {{\text{e}}^x}\sin x,{\text{ }}0 \leqslant x \leqslant \pi \).
The curvature at any point \((x,{\text{ }}y)\) on a graph is defined as \(\kappa = \frac{{\left| {\frac{{{{\text{d}}^2}y}}{{{\text{d}}{x^2}}}} \right|}}{{{{\left( {1 + {{\left( {\frac{{{\text{d}}y}}{{{\text{d}}x}}} \right)}^2}} \right)}^{\frac{3}{2}}}}}\).
Find an expression for \(\frac{{{\text{d}}y}}{{{\text{d}}x}}\).
Show that \(\frac{{{{\text{d}}^2}y}}{{{\text{d}}{x^2}}} = 2{{\text{e}}^x}\cos x\).
Show that the function \(f\) has a local maximum value when \(x = \frac{{3\pi }}{4}\).
Find the \(x\)-coordinate of the point of inflexion of the graph of \(f\).
Sketch the graph of \(f\), clearly indicating the position of the local maximum point, the point of inflexion and the axes intercepts.
Find the area of the region enclosed by the graph of \(f\) and the \(x\)-axis.
The curvature at any point \((x,{\text{ }}y)\) on a graph is defined as \(\kappa = \frac{{\left| {\frac{{{{\text{d}}^2}y}}{{{\text{d}}{x^2}}}} \right|}}{{{{\left( {1 + {{\left( {\frac{{{\text{d}}y}}{{{\text{d}}x}}} \right)}^2}} \right)}^{\frac{3}{2}}}}}\).
Find the value of the curvature of the graph of \(f\) at the local maximum point.
Find the value \(\kappa \) for \(x = \frac{\pi }{2}\) and comment on its meaning with respect to the shape of the graph.
▶️Answer/Explanation
Markscheme
\(\frac{{{\text{d}}y}}{{{\text{d}}x}} = {{\text{e}}^x}\sin x + {{\text{e}}^x}\cos x{\text{ }}\left( { = {{\text{e}}^x}(\sin x + \cos x)} \right)\) M1A1
[2 marks]
\(\frac{{{{\text{d}}^2}y}}{{{\text{d}}{x^2}}} = {{\text{e}}^x}(\sin x + \cos x) + {{\text{e}}^x}(\cos x – \sin x)\) M1A1
\( = 2{{\text{e}}^x}\cos x\) AG
[2 marks]
\(\frac{{{\text{d}}y}}{{{\text{d}}x}} = {{\text{e}}^{\frac{{3\pi }}{4}}}\left( {\sin \frac{{3\pi }}{4} + \cos \frac{{3\pi }}{4}} \right) = 0\) R1
\(\frac{{{{\text{d}}^2}y}}{{{\text{d}}{x^2}}} = 2{{\text{e}}^{\frac{{3\pi }}{4}}}\cos \frac{{3\pi }}{4} < 0\) R1
hence maximum at \(x = \frac{{3\pi }}{4}\) AG
[2 marks]
\(\frac{{{{\text{d}}^2}y}}{{{\text{d}}{x^2}}} = 0 \Rightarrow 2{{\text{e}}^x}\cos x = 0\) M1
\( \Rightarrow x = \frac{\pi }{2}\) A1
Note: Award M1A0 if extra zeros are seen.
[2 marks]
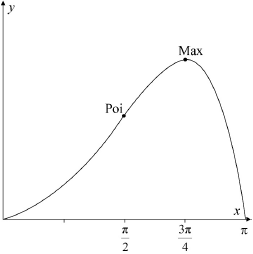
correct shape and correct domain A1
max at \(x = \frac{{3\pi }}{4}\), point of inflexion at \(x = \frac{\pi }{2}\) A1
zeros at \(x = 0\) and \(x = \pi \) A1
Note: Penalize incorrect domain with first A mark; allow FT from (d) on extra points of inflexion.
[3 marks]
EITHER
\(\int_0^x {{{\text{e}}^x}\sin x{\text{d}}x = [{{\text{e}}^x}\sin x]_0^\pi – \int_0^\pi {{{\text{e}}^x}\cos x{\text{d}}x} } \) M1A1
\(\int_0^\pi {{{\text{e}}^x}\sin x{\text{d}}x = [{{\text{e}}^x}\sin x]_0^\pi – \left( {[{{\text{e}}^x}\cos x]_0^x + \int_0^\pi {{{\text{e}}^x}\sin x{\text{d}}x} } \right)} \) A1
OR
\(\int_0^\pi {{{\text{e}}^x}\sin x{\text{d}}x = [ – {{\text{e}}^x}\cos x]_0^\pi + \int_0^\pi {{{\text{e}}^x}\cos x{\text{d}}x} } \) M1A1
\(\int_0^\pi {{{\text{e}}^x}\sin x{\text{d}}x = [ – {{\text{e}}^x}\cos x]} _0^\pi + \left( {[{{\text{e}}^x}\sin x]_0^\pi – \int_0^\pi {{{\text{e}}^x}\sin x{\text{d}}x} } \right)\) A1
THEN
\(\int_0^\pi {{{\text{e}}^x}\sin x{\text{d}}x = \frac{1}{2}\left( {[{{\text{e}}^x}\sin x]_0^x – [{{\text{e}}^x}\cos x]_0^x} \right)} \) M1A1
\(\int_0^\pi {{{\text{e}}^x}\sin x{\text{d}}x = \frac{1}{2}({{\text{e}}^x} + 1)} \) A1
[6 marks]
\(\frac{{{\text{d}}y}}{{{\text{d}}x}} = 0\) (A1)
\(\frac{{{d^2}y}}{{d{x^2}}} = 2{e^{\frac{{3\pi }}{4}}}\cos \frac{{3\pi }}{4} = – \sqrt 2 {e^{\frac{{3\pi }}{4}}}\) (A1)
\(\kappa = \frac{{\left| { – \sqrt 2 {{\text{e}}^{\frac{{3\pi }}{4}}}} \right|}}{1} = \sqrt 2 {{\text{e}}^{\frac{{3\pi }}{4}}}\) A1
[3 marks]
\(\kappa = 0\) A1
the graph is approximated by a straight line R1
[2 marks]
Examiners report
[N/A]
[N/A]
[N/A]
[N/A]
[N/A]
[N/A]
[N/A]
[N/A]
Question
Let \(y = {{\text{e}}^x}\sin x\).
Consider the function \(f\) defined by \(f(x) = {{\text{e}}^x}\sin x,{\text{ }}0 \leqslant x \leqslant \pi \).
The curvature at any point \((x,{\text{ }}y)\) on a graph is defined as \(\kappa = \frac{{\left| {\frac{{{{\text{d}}^2}y}}{{{\text{d}}{x^2}}}} \right|}}{{{{\left( {1 + {{\left( {\frac{{{\text{d}}y}}{{{\text{d}}x}}} \right)}^2}} \right)}^{\frac{3}{2}}}}}\).
Find an expression for \(\frac{{{\text{d}}y}}{{{\text{d}}x}}\).
Show that \(\frac{{{{\text{d}}^2}y}}{{{\text{d}}{x^2}}} = 2{{\text{e}}^x}\cos x\).
Show that the function \(f\) has a local maximum value when \(x = \frac{{3\pi }}{4}\).
Find the \(x\)-coordinate of the point of inflexion of the graph of \(f\).
Sketch the graph of \(f\), clearly indicating the position of the local maximum point, the point of inflexion and the axes intercepts.
Find the area of the region enclosed by the graph of \(f\) and the \(x\)-axis.
The curvature at any point \((x,{\text{ }}y)\) on a graph is defined as \(\kappa = \frac{{\left| {\frac{{{{\text{d}}^2}y}}{{{\text{d}}{x^2}}}} \right|}}{{{{\left( {1 + {{\left( {\frac{{{\text{d}}y}}{{{\text{d}}x}}} \right)}^2}} \right)}^{\frac{3}{2}}}}}\).
Find the value of the curvature of the graph of \(f\) at the local maximum point.
Find the value \(\kappa \) for \(x = \frac{\pi }{2}\) and comment on its meaning with respect to the shape of the graph.
▶️Answer/Explanation
Markscheme
\(\frac{{{\text{d}}y}}{{{\text{d}}x}} = {{\text{e}}^x}\sin x + {{\text{e}}^x}\cos x{\text{ }}\left( { = {{\text{e}}^x}(\sin x + \cos x)} \right)\) M1A1
[2 marks]
\(\frac{{{{\text{d}}^2}y}}{{{\text{d}}{x^2}}} = {{\text{e}}^x}(\sin x + \cos x) + {{\text{e}}^x}(\cos x – \sin x)\) M1A1
\( = 2{{\text{e}}^x}\cos x\) AG
[2 marks]
\(\frac{{{\text{d}}y}}{{{\text{d}}x}} = {{\text{e}}^{\frac{{3\pi }}{4}}}\left( {\sin \frac{{3\pi }}{4} + \cos \frac{{3\pi }}{4}} \right) = 0\) R1
\(\frac{{{{\text{d}}^2}y}}{{{\text{d}}{x^2}}} = 2{{\text{e}}^{\frac{{3\pi }}{4}}}\cos \frac{{3\pi }}{4} < 0\) R1
hence maximum at \(x = \frac{{3\pi }}{4}\) AG
[2 marks]
\(\frac{{{{\text{d}}^2}y}}{{{\text{d}}{x^2}}} = 0 \Rightarrow 2{{\text{e}}^x}\cos x = 0\) M1
\( \Rightarrow x = \frac{\pi }{2}\) A1
Note: Award M1A0 if extra zeros are seen.
[2 marks]

correct shape and correct domain A1
max at \(x = \frac{{3\pi }}{4}\), point of inflexion at \(x = \frac{\pi }{2}\) A1
zeros at \(x = 0\) and \(x = \pi \) A1
Note: Penalize incorrect domain with first A mark; allow FT from (d) on extra points of inflexion.
[3 marks]
EITHER
\(\int_0^x {{{\text{e}}^x}\sin x{\text{d}}x = [{{\text{e}}^x}\sin x]_0^\pi – \int_0^\pi {{{\text{e}}^x}\cos x{\text{d}}x} } \) M1A1
\(\int_0^\pi {{{\text{e}}^x}\sin x{\text{d}}x = [{{\text{e}}^x}\sin x]_0^\pi – \left( {[{{\text{e}}^x}\cos x]_0^x + \int_0^\pi {{{\text{e}}^x}\sin x{\text{d}}x} } \right)} \) A1
OR
\(\int_0^\pi {{{\text{e}}^x}\sin x{\text{d}}x = [ – {{\text{e}}^x}\cos x]_0^\pi + \int_0^\pi {{{\text{e}}^x}\cos x{\text{d}}x} } \) M1A1
\(\int_0^\pi {{{\text{e}}^x}\sin x{\text{d}}x = [ – {{\text{e}}^x}\cos x]} _0^\pi + \left( {[{{\text{e}}^x}\sin x]_0^\pi – \int_0^\pi {{{\text{e}}^x}\sin x{\text{d}}x} } \right)\) A1
THEN
\(\int_0^\pi {{{\text{e}}^x}\sin x{\text{d}}x = \frac{1}{2}\left( {[{{\text{e}}^x}\sin x]_0^x – [{{\text{e}}^x}\cos x]_0^x} \right)} \) M1A1
\(\int_0^\pi {{{\text{e}}^x}\sin x{\text{d}}x = \frac{1}{2}({{\text{e}}^x} + 1)} \) A1
[6 marks]
\(\frac{{{\text{d}}y}}{{{\text{d}}x}} = 0\) (A1)
\(\frac{{{d^2}y}}{{d{x^2}}} = 2{e^{\frac{{3\pi }}{4}}}\cos \frac{{3\pi }}{4} = – \sqrt 2 {e^{\frac{{3\pi }}{4}}}\) (A1)
\(\kappa = \frac{{\left| { – \sqrt 2 {{\text{e}}^{\frac{{3\pi }}{4}}}} \right|}}{1} = \sqrt 2 {{\text{e}}^{\frac{{3\pi }}{4}}}\) A1
[3 marks]
\(\kappa = 0\) A1
the graph is approximated by a straight line R1
[2 marks]
Examiners report
[N/A]
[N/A]
[N/A]
[N/A]
[N/A]
[N/A]
[N/A]
[N/A]
Question
Consider the function \(f\) defined by \(f(x) = {x^2} – {a^2},{\text{ }}x \in \mathbb{R}\) where \(a\) is a positive constant.
The function \(g\) is defined by \(g(x) = x\sqrt {f(x)} \) for \(\left| x \right| > a\).
Showing any \(x\) and \(y\) intercepts, any maximum or minimum points and any asymptotes, sketch the following curves on separate axes.
\(y = f(x)\);
Showing any \(x\) and \(y\) intercepts, any maximum or minimum points and any asymptotes, sketch the following curves on separate axes.
\(y = \frac{1}{{f(x)}}\);
Showing any \(x\) and \(y\) intercepts, any maximum or minimum points and any asymptotes, sketch the following curves on separate axes.
\(y = \left| {\frac{1}{{f(x)}}} \right|\).
Find \(\int {f(x)\cos x{\text{d}}x} \).
By finding \(g'(x)\) explain why \(g\) is an increasing function.
▶️Answer/Explanation
Markscheme
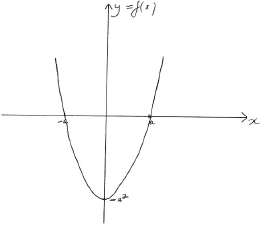
A1 for correct shape
A1 for correct \(x\) and \(y\) intercepts and minimum point
[2 marks]
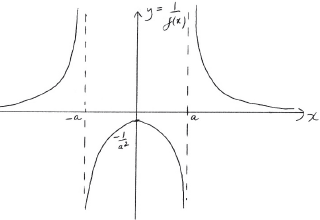
A1 for correct shape
A1 for correct vertical asymptotes
A1 for correct implied horizontal asymptote
A1 for correct maximum point
[??? marks]

A1 for reflecting negative branch from (ii) in the \(x\)-axis
A1 for correctly labelled minimum point
[2 marks]
EITHER
attempt at integration by parts (M1)
\(\int {({x^2} – {a^2})\cos x{\text{d}}x = ({x^2} – {a^2})\sin x – \int {2x\sin x{\text{d}}x} } \) A1A1
\( = ({x^2} – {a^2})\sin x – 2\left[ { – x\cos x + \int {\cos x{\text{d}}x} } \right]\) A1
\( = ({x^2} – {a^2})\sin x + 2x\cos – 2\sin x + c\) A1
OR
\(\int {({x^2} – {a^2})\cos x{\text{d}}x = \int {{x^2}\cos x{\text{d}}x – \int {{a^2}\cos x{\text{d}}x} } } \)
attempt at integration by parts (M1)
\(\int {{x^2}\cos x{\text{d}}x = {x^2}\sin x – \int {2x\sin x{\text{d}}x} } \) A1A1
\( = {x^2}\sin x – 2\left[ { – x\cos x + \int {\cos x{\text{d}}x} } \right]\) A1
\( = {x^2}\sin x + 2x\cos x – 2\sin x\)
\( – \int {{a^2}\cos x{\text{d}}x = – {a^2}\sin x} \)
\(\int {({x^2} – {a^2})\cos x{\text{d}}x = ({x^2} – {a^2})\sin x + 2x\cos x – 2\sin x + c} \) A1
[5 marks]
\(g(x) = x{({x^2} – {a^2})^{\frac{1}{2}}}\)
\(g'(x) = {({x^2} – {a^2})^{\frac{1}{2}}} + \frac{1}{2}x{({x^2} – {a^2})^{ – \frac{1}{2}}}(2x)\) M1A1A1
Note: Method mark is for differentiating the product. Award A1 for each correct term.
\(g'(x) = {({x^2} – {a^2})^{\frac{1}{2}}} + {x^2}{({x^2} – {a^2})^{ – \frac{1}{2}}}\)
both parts of the expression are positive hence \(g'(x)\) is positive R1
and therefore \(g\) is an increasing function (for \(\left| x \right| > a\)) AG
[4 marks]
Examiners report
[N/A]
[N/A]
[N/A]
[N/A]
[N/A]
Question
Consider the function \({f_n}(x) = (\cos 2x)(\cos 4x) \ldots (\cos {2^n}x),{\text{ }}n \in {\mathbb{Z}^ + }\).
Determine whether \({f_n}\) is an odd or even function, justifying your answer.
By using mathematical induction, prove that
\({f_n}(x) = \frac{{\sin {2^{n + 1}}x}}{{{2^n}\sin 2x}},{\text{ }}x \ne \frac{{m\pi }}{2}\) where \(m \in \mathbb{Z}\).
Hence or otherwise, find an expression for the derivative of \({f_n}(x)\) with respect to \(x\).
Show that, for \(n > 1\), the equation of the tangent to the curve \(y = {f_n}(x)\) at \(x = \frac{\pi }{4}\) is \(4x – 2y – \pi = 0\).
▶️Answer/Explanation
Markscheme
even function A1
since \(\cos kx = \cos ( – kx)\) and \({f_n}(x)\) is a product of even functions R1
OR
even function A1
since \((\cos 2x)(\cos 4x) \ldots = \left( {\cos ( – 2x)} \right)\left( {\cos ( – 4x)} \right) \ldots \) R1
Note: Do not award A0R1.
[2 marks]
consider the case \(n = 1\)
\(\frac{{\sin 4x}}{{2\sin 2x}} = \frac{{2\sin 2x\cos 2x}}{{2\sin 2x}} = \cos 2x\) M1
hence true for \(n = 1\) R1
assume true for \(n = k\), ie, \((\cos 2x)(\cos 4x) \ldots (\cos {2^k}x) = \frac{{\sin {2^{k + 1}}x}}{{{2^k}\sin 2x}}\) M1
Note: Do not award M1 for “let \(n = k\)” or “assume \(n = k\)” or equivalent.
consider \(n = k + 1\):
\({f_{k + 1}}(x) = {f_k}(x)(\cos {2^{k + 1}}x)\) (M1)
\( = \frac{{\sin {2^{k + 1}}x}}{{{2^k}\sin 2x}}\cos {2^{k + 1}}x\) A1
\( = \frac{{2\sin {2^{k + 1}}x\cos {2^{k + 1}}x}}{{{2^{k + 1}}\sin 2x}}\) A1
\( = \frac{{\sin {2^{k + 2}}x}}{{{2^{k + 1}}\sin 2x}}\) A1
so \(n = 1\) true and \(n = k\) true \( \Rightarrow n = k + 1\) true. Hence true for all \(n \in {\mathbb{Z}^ + }\) R1
Note: To obtain the final R1, all the previous M marks must have been awarded.
[8 marks]
attempt to use \(f’ = \frac{{vu’ – uv’}}{{{v^2}}}\) (or correct product rule) M1
\({f’_n}(x) = \frac{{({2^n}\sin 2x)({2^{n + 1}}\cos {2^{n + 1}}x) – (\sin {2^{n + 1}}x)({2^{n + 1}}\cos 2x)}}{{{{({2^n}\sin 2x)}^2}}}\) A1A1
Note: Award A1 for correct numerator and A1 for correct denominator.
[3 marks]
\({f’_n}\left( {\frac{\pi }{4}} \right) = \frac{{\left( {{2^n}\sin \frac{\pi }{2}} \right)\left( {{2^{n + 1}}\cos {2^{n + 1}}\frac{\pi }{4}} \right) – \left( {\sin {2^{n + 1}}\frac{\pi }{4}} \right)\left( {{2^{n + 1}}\cos \frac{\pi }{2}} \right)}}{{{{\left( {{2^n}\sin \frac{\pi }{2}} \right)}^2}}}\) (M1)(A1)
\({f’_n}\left( {\frac{\pi }{4}} \right) = \frac{{({2^n})\left( {{2^{n + 1}}\cos {2^{n + 1}}\frac{\pi }{4}} \right)}}{{{{({2^n})}^2}}}\) (A1)
\( = 2\cos {2^{n + 1}}\frac{\pi }{4}{\text{ }}( = 2\cos {2^{n – 1}}\pi )\) A1
\({f’_n}\left( {\frac{\pi }{4}} \right) = 2\) A1
\({f_n}\left( {\frac{\pi }{4}} \right) = 0\) A1
Note: This A mark is independent from the previous marks.
\(y = 2\left( {x – \frac{\pi }{4}} \right)\) M1A1
\(4x – 2y – \pi = 0\) AG
[8 marks]
Examiners report
[N/A]
[N/A]
[N/A]
[N/A]
Question
The function f is defined by
\[f(x) = \left\{ {\begin{array}{*{20}{c}}
{2x – 1,}&{x \leqslant 2} \\
{a{x^2} + bx – 5,}&{2 < x < 3}
\end{array}} \right.\]
where a , \(b \in \mathbb{R}\) .
Given that f and its derivative, \(f’\) , are continuous for all values in the domain of f , find the values of a and b .
Show that f is a one-to-one function.
Obtain expressions for the inverse function \({f^{ – 1}}\) and state their domains.
▶️Answer/Explanation
Markscheme
f continuous \( \Rightarrow \mathop {\lim }\limits_{x \to {2^ – }} f(x) = \mathop {\lim }\limits_{x \to {2^ \div }} f(x)\) M1
\(4a + 2b = 8\) A1
\(f'(x) = \left\{ {\begin{array}{*{20}{c}}
{2,}&{x < 2} \\
{2ax + b,}&{2 < x < 3}
\end{array}} \right.\) A1
\(f'{\text{ continuous}} \Rightarrow \mathop {\lim }\limits_{x \to {2^ – }} f'(x) = \mathop {\lim }\limits_{x \to {2^ \div }} f'(x)\)
\(4a + b = 2\) A1
solve simultaneously M1
to obtain a = –1 and b = 6 A1
[6 marks]
for \(x \leqslant 2,{\text{ }}f'(x) = 2 > 0\) A1
for \(2 < x < 3,{\text{ }}f'(x) = – 2x + 6 > 0\) A1
since \(f'(x) > 0\) for all values in the domain of f , f is increasing R1
therefore one-to-one AG
[3 marks]
\(x = 2y – 1 \Rightarrow y = \frac{{x + 1}}{2}\) M1
\(x = – {y^2} + 6y – 5 \Rightarrow {y^2} – 6y + x + 5 = 0\) M1
\(y = 3 \pm \sqrt {4 – x} \)
therefore
\({f^{ – 1}}(x) = \left\{ {\begin{array}{*{20}{c}}
{\frac{{x + 1}}{2},}&{x \leqslant 3} \\
{3 – \sqrt {4 – x} ,}&{3 < x < 4}
\end{array}} \right.\) A1A1A1
Note: Award A1 for the first line and A1A1 for the second line.
[5 marks]
Examiners report
[N/A]
[N/A]
[N/A]
