IBDP Maths analysis and approaches Topic: AHL 3.13 :Algebraic and geometric approaches to magnitude of a vector, |v| HL Paper 1

IB DP PhysicsIB DP Maths IB DP ChemistryIB DP Biology

Question

The three vectors \(\boldsymbol{a}\), \(\boldsymbol{b}\) and \(\boldsymbol{c}\) are given by\[{\boldsymbol{a}} = \left( {\begin{array}{*{20}{c}}
{2y} \\
{ – 3x} \\
{2x}
\end{array}} \right),{\text{ }}{\boldsymbol{b}}{\text{ }} = \left( {\begin{array}{*{20}{c}}
{4x} \\
y \\
{3 – x}
\end{array}} \right),{\text{ }}{\boldsymbol{c}}{\text{ }} = \left( {\begin{array}{*{20}{c}}
4 \\
{ – 7} \\
6
\end{array}} \right){\text{ where }}x,y \in \mathbb{R}{\text{ }}{\text{.}}\]

(a) If a + 2b − c = 0, find the value of x and of y.

(b) Find the exact value of \(|\)a + 2b\(|\).

Answer/Explanation

Markscheme

(a) \(2y + 8x = 4\) M1

\( – 3x + 2y = – 7\) A1

\(2x + 6 – 2x = 6\)

Note: Award M1 for attempt at components, A1 for two correct equations.

No penalty for not checking the third equation.

solving : x = 1, y = –2 A1

(b) \(|\)a + 2b\(| = \left| {\left( {\begin{array}{*{20}{c}}
{ – 4} \\
{ – 3} \\
2
\end{array}} \right) + 2\left( {\begin{array}{*{20}{c}}
4 \\
{ – 2} \\
2
\end{array}} \right)} \right|\)

\( = \left| {\left( {\begin{array}{*{20}{c}}
4 \\
{ – 7} \\
6
\end{array}} \right)} \right|\)

\( \Rightarrow |\)a + 2b\(|\) \( = \sqrt {{4^2} + {{( – 7)}^2} + {6^2}} \) (M1)

\( = \sqrt {101} \) A1

[5 marks]

Examiners report

The majority of candidates understood what was required in part (a) of this question and gained the correct answer. Most candidates were able to do part (b) but few realised that they did not have to calculate \(|\)a + 2b\(|\) as this is \(|\)c\(|\). Many candidates lost time on this question.

Question

Let \(\alpha \) be the angle between the unit vectors a and b, where \(0 \leqslant \alpha \leqslant \pi \).

(a) Express \(|\)a − b\(|\) and \(|\)a + b\(|\) in terms of \(\alpha \).

(b) Hence determine the value of \(\cos \alpha \) for which \(|\)a + b\(|\) = 3 \(|\)a − b\(|\).

Answer/Explanation

Markscheme

METHOD 1

(a) \(|\)a – b\(|\) = \(\sqrt {{{\left| a \right|}^2} + {{\left| b \right|}^2} – 2\left| a \right|\left| b \right|\cos \alpha } \) M1

\( = \sqrt {2 – 2\cos \alpha } \) A1

\(|\)a + b\(|\) = \(\sqrt {{{\left| a \right|}^2} + {{\left| b \right|}^2} – 2\left| a \right|\left| b \right|\cos (\pi – \alpha )} \)

\( = \sqrt {2 + 2\cos \alpha } \) A1

Note: Accept the use of a, b for \(|\)a\(|\), \(|\)b\(|\).

(b) \( = \sqrt {2 + 2\cos \alpha } = 3\sqrt {2 – 2\cos \alpha } \) M1

\(\cos \alpha = \frac{4}{5}\) A1

METHOD 2

(a) \(|\)a – b\(|\) = \(2\sin \frac{\alpha}{2}\) M1A1

\(|\)a + b\(|\) = \(2\sin \left( {\frac{\pi }{2} – \frac{\alpha}{2}} \right) = 2\cos \frac{\alpha}{2}\) A1

Note: Accept the use of a, b for \(|\)a \(|\), \(|\)b\(|\).

(b) \(2\cos \frac{\alpha}{2} = 6\sin \frac{\alpha }{2}\)

\(\tan \frac{\alpha }{2} = \frac{1}{3} \Rightarrow {\cos ^2}\frac{\alpha }{2} = \frac{9}{{10}}\) M1

\(\cos \alpha = 2{\cos ^2}\frac{\alpha }{2} – 1 = \frac{4}{5}\) A1

[5 marks]

Examiners report

To solve this problem, candidates had to know either that (a + b)(a + b) = \(|\)a + b\({|^2}\) or that the diagonals of a parallelogram whose sides are a and b represent the vectors a + b and a – b. It was clear from the scripts that many candidates were unaware of either result and were therefore unable to make any progress in this question.

Question

In the diagram below, [AB] is a diameter of the circle with centre O. Point C is on the circumference of the circle. Let \(\overrightarrow {{\text{OB}}} = \boldsymbol{b} \) and \(\overrightarrow {{\text{OC}}} = \boldsymbol{c}\) .

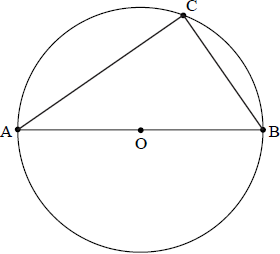
Find an expression for \(\overrightarrow {{\text{CB}}} \) and for \(\overrightarrow {{\text{AC}}} \) in terms of \(\boldsymbol{b}\) and \(\boldsymbol{c}\) .

[2]

Hence prove that \({\rm{A\hat CB}}\) is a right angle.

[3]

Answer/Explanation

Markscheme

\(\overrightarrow {{\text{CB}}} = \boldsymbol{b} – \boldsymbol{c}\) , \(\overrightarrow {{\text{AC}}} = \boldsymbol{b} + \boldsymbol{c}\) A1A1

Note: Condone absence of vector notation in (a).

[2 marks]

\(\overrightarrow {{\text{AC}}} \cdot \overrightarrow {{\text{CB}}} = \)(b + c)\( \cdot \)(b – c) M1

= \(|\)b\({|^2}\) – \(|\)c\({|^2}\) A1

= 0 since \(|\)b\(|\) = \(|\)c\(|\) R1

Note: Only award the A1 and R1 if working indicates that they understand that they are working with vectors.

so \(\overrightarrow {{\text{AC}}} \) is perpendicular to \(\overrightarrow {{\text{CB}}} \) i.e. \({\rm{A\hat CB}}\) is a right angle AG

[3 marks]

Examiners report

Most candidates were able to find the expressions for the two vectors although a number were not able to do this. Most then tried to use Pythagoras’ theorem and confused scalars and vectors. There were few correct responses to the second part. Candidates did not seem to be able to use the algebra of vectors comfortably.

Question

Show that the points \({\text{O}}(0,{\text{ }}0,{\text{ }}0)\), \({\text{ A}}(6,{\text{ }}0,{\text{ }}0)\), \({\text{B}}({6,{\text{ }}- \sqrt {24} ,{\text{ }}\sqrt {12} })\), \({\text{C}}({0,{\text{ }}- \sqrt {24} ,{\text{ }}\sqrt {12}})\) form a square.

[3]

Find the coordinates of M, the mid-point of [OB].

[1]

Show that an equation of the plane \({\mathit{\Pi }}\), containing the square OABC, is \(y + \sqrt 2 z = 0\).

[3]

Find a vector equation of the line \(L\), through M, perpendicular to the plane \({\mathit{\Pi }}\).

[3]

Find the coordinates of D, the point of intersection of the line \(L\) with the plane whose equation is \(y = 0\).

[3]

Find the coordinates of E, the reflection of the point D in the plane \({\mathit{\Pi }}\).

[3]

(i) Find the angle \({\rm{O\hat DA}}\).

(ii) State what this tells you about the solid OABCDE.

[6]

Answer/Explanation

Markscheme

\(\left| {\overrightarrow {{\text{OA}}} } \right| = \left| {\overrightarrow {{\text{CB}}} } \right| = \left| {\overrightarrow {{\text{OC}}} } \right| = \left| {\overrightarrow {{\text{AB}}} } \right| = 6\) (therefore a rhombus) A1A1

Note: Award A1 for two correct lengths, A2 for all four.

Note: Award A1A0 for \(\overrightarrow {{\rm{OA}}} = \overrightarrow {{\rm{CB}}} = \left( \begin{array}{l}6\\0\\0\end{array} \right){\rm{ or \,\,} } \overrightarrow {{\rm{OC}}} = \overrightarrow {A{\rm{B}}} = \left( \begin{array}{c}0\\ – \sqrt {24} \\\sqrt {12} \end{array} \right)\) if no magnitudes are shown.

\(\overrightarrow {{\rm{OA}}}\,\, {\rm{ g}}\overrightarrow {{\rm{OC}}} = \left( \begin{array}{l}6\\0\\0\end{array} \right){\rm{g}}\left( \begin{array}{c}0\\ – \sqrt {24} \\\sqrt {12} \end{array} \right) = 0 \) (therefore a square) A1

Note: Other arguments are possible with a minimum of three conditions.

[3 marks]

\({\text{M}}\left( {3,{\text{ }} – \frac{{\sqrt {24} }}{2},{\text{ }}\frac{{\sqrt {12} }}{2}} \right)\left( { = \left( {3,{\text{ }} – \sqrt 6 ,{\text{ }}\sqrt 3 } \right)} \right)\) A1

[1 mark]

METHOD 1

\(\overrightarrow {{\text{OA}}} \times \overrightarrow {{\text{OC}}} = \)\(\left( \begin{array}{l}6\\0\\0\end{array} \right) \times \left( \begin{array}{c}0\\ – \sqrt {24} \\\sqrt {12} \end{array} \right) = \left( \begin{array}{c}0\\ – 6\sqrt {12} \\ – 6\sqrt {24} \end{array} \right)\left( { = \left( \begin{array}{c}0\\ – 12\sqrt 3 \\ – 12\sqrt 6 \end{array} \right)} \right)\) M1A1

Note: Candidates may use other pairs of vectors.

equation of plane is \( – 6\sqrt {12} y – 6\sqrt {24} z = d\)

any valid method showing that \(d = 0\) M1

\(\mathit{\Pi} :y+\sqrt{2z}=0\) AG

METHOD 2

equation of plane is \(ax + by + cz = d\)

substituting O to find \(d = 0\) (M1)

substituting two points (A, B, C or M) M1

\(6a = 0,{\text{ }} – \sqrt {24} b + \sqrt {12} c = 0\) A1

\(\mathit{\Pi} :y+\sqrt{2z}=0\) AG

[3 marks]

\(\boldsymbol{r} = \left( \begin{array}{c}3\\ – \sqrt 6 \\\sqrt 3 \end{array} \right) + \lambda \left( \begin{array}{l}0\\1\\\sqrt 2 \end{array} \right)\) A1A1A1

Note: Award A1 for r = , A1A1 for two correct vectors.

[3 marks]

Using \(y = 0\) to find \(\lambda \) M1

Substitute their \(\lambda \) into their equation from part (d) M1

D has coordinates \(\left( {{\text{3, 0, 3}}\sqrt 3 } \right)\) A1

[3 marks]

\(\lambda \) for point E is the negative of the \(\lambda \) for point D (M1)

Note: Other possible methods may be seen.

E has coordinates \(\left( {{\text{3, }} – 2\sqrt 6 ,{\text{ }} – \sqrt 3 } \right)\) A1A1

Note: Award A1 for each of the y and z coordinates.

[3 marks]

(i) \(\overrightarrow {{\text{DA}}} {\text{ g}}\overrightarrow {{\text{DO}}} = \)\(\left( \begin{array}{c}3\\0\\ – 3\sqrt 3 \end{array} \right){\rm{g}}\left( \begin{array}{c} – 3\\0\\ – 3\sqrt 3 \end{array} \right) = 18\) M1A1

\(\cos {\rm{O\hat DA}} = \frac{{18}}{{\sqrt {36} \sqrt {36} }} = \frac{1}{2}\) M1

hence \({\rm{O\hat DA}} = 60^\circ \) A1

Note: Accept method showing OAD is equilateral.

(ii) OABCDE is a regular octahedron (accept equivalent description) A2

Note: A2 for saying it is made up of 8 equilateral triangles

Award A1 for two pyramids, A1 for equilateral triangles.

(can be either stated or shown in a sketch – but there must be clear indication the triangles are equilateral)

[6 marks]

Examiners report

[N/A]

Question

[3]

Find the coordinates of M, the mid-point of [OB].

[1]

Show that an equation of the plane \({\mathit{\Pi }}\), containing the square OABC, is \(y + \sqrt 2 z = 0\).

[3]

Find a vector equation of the line \(L\), through M, perpendicular to the plane \({\mathit{\Pi }}\).

[3]

Find the coordinates of D, the point of intersection of the line \(L\) with the plane whose equation is \(y = 0\).

[3]

Find the coordinates of E, the reflection of the point D in the plane \({\mathit{\Pi }}\).

[3]

(i) Find the angle \({\rm{O\hat DA}}\).

(ii) State what this tells you about the solid OABCDE.

[6]

Answer/Explanation

Markscheme

Note: Award A1 for two correct lengths, A2 for all four.

Note: Other arguments are possible with a minimum of three conditions.

[3 marks]

\({\text{M}}\left( {3,{\text{ }} – \frac{{\sqrt {24} }}{2},{\text{ }}\frac{{\sqrt {12} }}{2}} \right)\left( { = \left( {3,{\text{ }} – \sqrt 6 ,{\text{ }}\sqrt 3 } \right)} \right)\) A1

[1 mark]

METHOD 1

Note: Candidates may use other pairs of vectors.

equation of plane is \( – 6\sqrt {12} y – 6\sqrt {24} z = d\)

any valid method showing that \(d = 0\) M1

\(\mathit{\Pi} :y+\sqrt{2z}=0\) AG

METHOD 2

equation of plane is \(ax + by + cz = d\)

substituting O to find \(d = 0\) (M1)

substituting two points (A, B, C or M) M1

\(6a = 0,{\text{ }} – \sqrt {24} b + \sqrt {12} c = 0\) A1

\(\mathit{\Pi} :y+\sqrt{2z}=0\) AG

[3 marks]

\(\boldsymbol{r} = \left( \begin{array}{c}3\\ – \sqrt 6 \\\sqrt 3 \end{array} \right) + \lambda \left( \begin{array}{l}0\\1\\\sqrt 2 \end{array} \right)\) A1A1A1

Note: Award A1 for r = , A1A1 for two correct vectors.

[3 marks]

Using \(y = 0\) to find \(\lambda \) M1

Substitute their \(\lambda \) into their equation from part (d) M1

D has coordinates \(\left( {{\text{3, 0, 3}}\sqrt 3 } \right)\) A1

[3 marks]

\(\lambda \) for point E is the negative of the \(\lambda \) for point D (M1)

Note: Other possible methods may be seen.

E has coordinates \(\left( {{\text{3, }} – 2\sqrt 6 ,{\text{ }} – \sqrt 3 } \right)\) A1A1

Note: Award A1 for each of the y and z coordinates.

[3 marks]

\(\cos {\rm{O\hat DA}} = \frac{{18}}{{\sqrt {36} \sqrt {36} }} = \frac{1}{2}\) M1

hence \({\rm{O\hat DA}} = 60^\circ \) A1

Note: Accept method showing OAD is equilateral.

(ii) OABCDE is a regular octahedron (accept equivalent description) A2

Note: A2 for saying it is made up of 8 equilateral triangles

Award A1 for two pyramids, A1 for equilateral triangles.

(can be either stated or shown in a sketch – but there must be clear indication the triangles are equilateral)

[6 marks]

Examiners report

[N/A]

Question

PQRS is a rhombus. Given that \(\overrightarrow {{\text{PQ}}} = \) \(\boldsymbol{a}\) and \(\overrightarrow {{\text{QR}}} = \) \(\boldsymbol{b}\),

(a) express the vectors \(\overrightarrow {{\text{PR}}} \) and \(\overrightarrow {{\text{QS}}} \) in terms of \(\boldsymbol{a}\) and \(\boldsymbol{b}\);

(b) hence show that the diagonals in a rhombus intersect at right angles.

Answer/Explanation

Markscheme

(a) \(\overrightarrow {{\text{PR}}} = \) a + b A1

\(\overrightarrow {{\text{QS}}} = \) b − a A1

[2 marks]

(b) \(\overrightarrow {{\text{PR}}} \cdot \overrightarrow {{\text{QS}}} = \) (a + b) \( \cdot \) (b − a) M1

\( = |\)b\({|^2} – |\)a\({|^2}\) A1

for a rhombus \(|\)a\(| = |\)b\(|\) R1

hence \(|\)b\({|^2} – |\)a\({|^2} = 0\) A1

Note: Do not award the final A1 unless R1 is awarded.

hence the diagonals intersect at right angles AG

[4 marks]

Total [6 marks]

Examiners report

[N/A]

Question

A point \(P\), relative to an origin \(O\), has position vector \(\overrightarrow {{\text{OP}}} = \left( {\begin{array}{*{20}{c}} {1 + s} \\ {3 + 2s} \\ {1 – s} \end{array}} \right),{\text{ }}s \in \mathbb{R}\).

Find the minimum length of \(\overrightarrow {{\text{OP}}} \).

Answer/Explanation

Markscheme

METHOD 1

\(\left| {\overrightarrow {{\text{OP}}} } \right| = \sqrt {{{(1 + s)}^2} + {{(3 + 2s)}^2} + {{(1 – s)}^2}} \;\;\;\left( { = \sqrt {6{s^2} + 12s + 11} } \right)\) A1

Note: Award A1 if the square of the distance is found.

EITHER

attempt to differentiate: \(\frac{{\text{d}}}{{{\text{d}}s}}{\left| {\overrightarrow {{\text{OP}}} } \right|^2}\;\;\;( = 12s + 12)\) M1

attempting to solve \(\frac{{\text{d}}}{{{\text{d}}s}}{\left| {\overrightarrow {{\text{OP}}} } \right|^2} = 0\;\;\;{\text{for }}s\) (M1)

\(s = – 1\) (A1)

attempt to differentiate: \(\frac{{\text{d}}}{{{\text{d}}s}}\left| {\overrightarrow {{\text{OP}}} } \right|\;\;\;\left( { = \frac{{6s + 6}}{{\sqrt {6{s^2} + 12s + 11} }}} \right)\) M1

attempting to solve \(\frac{{\text{d}}}{{{\text{d}}s}}\left| {\overrightarrow {{\text{OP}}} } \right| = 0\;\;\;{\text{for }}s\) (M1)

\(s = – 1\) (A1)

attempt at completing the square: \(\left( {{{\left| {\overrightarrow {{\text{OP}}} } \right|}^2} = 6{{(s + 1)}^2} + 5} \right)\) M1

minimum value (M1)

occurs at \(s = – 1\) (A1)

THEN

the minimum length of \(\overrightarrow {{\text{OP}}} \) is \(\sqrt 5 \) A1

METHOD 2

the length of \(\overrightarrow {{\text{OP}}} \) is a minimum when \(\overrightarrow {{\text{OP}}} \) is perpendicular to \(\left( {\begin{array}{*{20}{c}} 1 \\ 2 \\ { – 1} \end{array}} \right)\) (R1)

\(\left( {\begin{array}{*{20}{c}} {1 + s} \\ {3 + 2s} \\ {1 – s} \end{array}} \right) \bullet \left( {\begin{array}{*{20}{c}} 1 \\ 2 \\ { – 1} \end{array}} \right) = 0\) A1

attempting to solve \(1 + s + 6 + 4s – 1 + s = 0\;\;\;(6s + 6 = 0)\;\;\;{\text{for }}s\) (M1)

\(s = – 1\) (A1)

\(\left| {\overrightarrow {{\text{OP}}} } \right| = \sqrt 5 \) (A1)

[5 marks]

Examiners report

Generally well done. But there was a significant minority who didn’t realise that they had to use calculus or completion of squares to minimise the length. Trying random values of \(s\) gained no marks. A number of candidates wasted time showing that their answer gave a minimum rather than a maximum value of the length.

Question

O, A, B and C are distinct points such that \(\overrightarrow {{\text{OA}}} = \) a, \(\overrightarrow {{\text{OB}}} = \) b and \(\overrightarrow {{\text{OC}}} = \) c.

It is given that c is perpendicular to \(\overrightarrow {{\text{AB}}} \) and b is perpendicular to \(\overrightarrow {{\text{AC}}} \).

Prove that a is perpendicular to \(\overrightarrow {{\text{BC}}} \).

Answer/Explanation

Markscheme

c \( \bullet \) (b \( – \) a) \( = 0\) M1

Note: Allow c \( \bullet \) \(\overrightarrow {{\text{AB}}} = 0\) or similar for M1.

c \( \bullet \) b \( = \) c \( \bullet \) a A1

b \( \bullet \) (c \( – \) a) \( = 0\)

b \( \bullet \) c \( = \) b \( \bullet \) a A1

c \( \bullet \) a \( = \) b \( \bullet \) a M1

(c \( – \) b) \( \bullet \) a \( = 0\) A1

hence a is perpendicular to \(\overrightarrow {{\text{BC}}} \) AG

Note: Only award the final A1 if a dot is used throughout to indicate scalar product.

Condone any lack of specific indication that the letters represent vectors.

[5 marks]

Examiners report

This was generally poorly done. The recent syllabus change refers to ‘proof of geometrical properties using vectors’ and this is clearly a topic candidates are not entirely clear with at the moment. Despite the question clearly being written as a vector question some students tried to use a geometrical approach, assuming it was two-dimensional. Many did not seem to realise that vectors being perpendicular implies that their scalar product is zero.

Question

In the following diagram, \(\overrightarrow {{\text{OA}}} \) = a, \(\overrightarrow {{\text{OB}}} \) = b. C is the midpoint of [OA] and \(\overrightarrow {{\text{OF}}} = \frac{1}{6}\overrightarrow {{\text{FB}}} \).

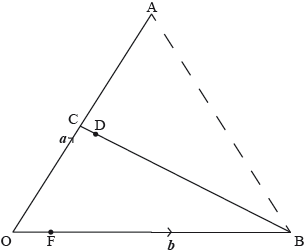
It is given also that \(\overrightarrow {{\text{AD}}} = \lambda \overrightarrow {{\text{AF}}} \) and \(\overrightarrow {{\text{CD}}} = \mu \overrightarrow {{\text{CB}}} \), where \(\lambda ,{\text{ }}\mu \in \mathbb{R}\).

Find, in terms of a and b \(\overrightarrow {{\text{OF}}} \).

[1]

a.i.

Find, in terms of a and b \(\overrightarrow {{\text{AF}}} \).

[2]

a.ii.

Find an expression for \(\overrightarrow {{\text{OD}}} \) in terms of a, b and \(\lambda \);

[2]

b.i.

Find an expression for \(\overrightarrow {{\text{OD}}} \) in terms of a, b and \(\mu \).

[2]

b.ii.

Show that \(\mu = \frac{1}{{13}}\), and find the value of \(\lambda \).

[4]

Deduce an expression for \(\overrightarrow {{\text{CD}}} \) in terms of a and b only.

[2]

Given that area \(\Delta {\text{OAB}} = k({\text{area }}\Delta {\text{CAD}})\), find the value of \(k\).

[5]

Answer/Explanation

Markscheme

\(\overrightarrow {{\text{OF}}} = \frac{1}{7}\)b A1

[1 mark]

a.i.

\(\overrightarrow {{\text{AF}}} = \overrightarrow {{\text{OF}}} – \overrightarrow {{\text{OA}}} \) (M1)

\( = \frac{1}{7}\)b – a A1

[2 marks]

a.ii.

\(\overrightarrow {{\text{OD}}} = \) a \( + \lambda \left( {\frac{1}{7}b -a} \right){\text{ }}\left( { = (1 – \lambda )a + \frac{\lambda }{7}b} \right)\) M1A1

[2 marks]

b.i.

\(\overrightarrow {{\text{OD}}} = \frac{1}{2}\) a \( + \mu \left( { – \frac{1}{2}a + b} \right){\text{ }}\left( { = \left( {\frac{1}{2} – \frac{\mu }{2}} \right)a + \mu b} \right)\) M1A1

[2 marks]

b.ii.

equating coefficients: M1

\(\frac{\lambda }{7} = \mu ,{\text{ }}1 – \lambda = \frac{{1 – \mu }}{2}\) A1

solving simultaneously: M1

\(\lambda = \frac{7}{{13}},{\text{ }}\mu = \frac{1}{{13}}\) A1AG

[4 marks]

\(\overrightarrow {{\text{CD}}} = \frac{1}{{13}}\overrightarrow {{\text{CB}}} \)

\( = \frac{1}{{13}}\left( {b – \frac{1}{2}a} \right){\text{ }}\left( { = – \frac{1}{{26}}a + \frac{1}{{13}}b} \right)\) M1A1

[2 marks]

METHOD 1

\({\text{area }}\Delta {\text{ACD}} = \frac{1}{2}{\text{CD}} \times {\text{AC}} \times \sin {\rm{A\hat CB}}\) (M1)

\({\text{area }}\Delta {\text{ACB}} = \frac{1}{2}{\text{CB}} \times {\text{AC}} \times \sin {\rm{A\hat CB}}\) (M1)

\({\text{ratio }}\frac{{{\text{area }}\Delta {\text{ACD}}}}{{{\text{area }}\Delta {\text{ACB}}}} = \frac{{{\text{CD}}}}{{{\text{CB}}}} = \frac{1}{{13}}\) A1

\(k = \frac{{{\text{area }}\Delta {\text{OAB}}}}{{{\text{area }}\Delta {\text{CAD}}}} = \frac{{13}}{{{\text{area }}\Delta {\text{CAB}}}} \times {\text{area }}\Delta {\text{OAB}}\) (M1)

\( = 13 \times 2 = 26\) A1

METHOD 2

\({\text{area }}\Delta {\text{OAB}} = \frac{1}{2}\left| {a \times b} \right|\) A1

\({\text{area }}\Delta {\text{CAD}} = \frac{1}{2}\left| {\overrightarrow {{\text{CA}}} \times \overrightarrow {{\text{CD}}} } \right|\) or \(\frac{1}{2}\left| {\overrightarrow {{\text{CA}}} \times \overrightarrow {{\text{AD}}} } \right|\) M1

\( = \frac{1}{2}\left| {\frac{1}{2}a \times \left( { – \frac{1}{{26}}a + \frac{1}{{13}}b} \right)} \right|\)

\( = \frac{1}{2}\left| {\frac{1}{2}a \times \left( { – \frac{1}{{26}}a} \right) + \frac{1}{2}a \times \frac{1}{{13}}b} \right|\) (M1)

\( = \frac{1}{2} \times \frac{1}{2} \times \frac{1}{{13}}\left| {a \times b} \right|{\text{ }}\left( { = \frac{1}{{52}}\left| {a \times b} \right|} \right)\) A1

\({\text{area }}\Delta {\text{OAB}} = k({\text{area }}\Delta {\text{CAD}})\)

\(\frac{1}{2}\left| {a \times b} \right| = k\frac{1}{{52}}\left| {a \times b} \right|\)

\(k = 26\) A1

[5 marks]

Examiners report

[N/A]

a.i.

[N/A]

a.ii.

[N/A]

b.i.

[N/A]

b.ii.

[N/A]

Question

Find, in terms of a and b \(\overrightarrow {{\text{OF}}} \).

[1]

a.i.

Find, in terms of a and b \(\overrightarrow {{\text{AF}}} \).

[2]

a.ii.

Find an expression for \(\overrightarrow {{\text{OD}}} \) in terms of a, b and \(\lambda \);

[2]

b.i.

Find an expression for \(\overrightarrow {{\text{OD}}} \) in terms of a, b and \(\mu \).

[2]

b.ii.

Show that \(\mu = \frac{1}{{13}}\), and find the value of \(\lambda \).

[4]

Deduce an expression for \(\overrightarrow {{\text{CD}}} \) in terms of a and b only.

[2]

Given that area \(\Delta {\text{OAB}} = k({\text{area }}\Delta {\text{CAD}})\), find the value of \(k\).

[5]

Answer/Explanation

Markscheme

\(\overrightarrow {{\text{OF}}} = \frac{1}{7}\)b A1

[1 mark]

a.i.

\(\overrightarrow {{\text{AF}}} = \overrightarrow {{\text{OF}}} – \overrightarrow {{\text{OA}}} \) (M1)

\( = \frac{1}{7}\)b – a A1

[2 marks]

a.ii.

\(\overrightarrow {{\text{OD}}} = \) a \( + \lambda \left( {\frac{1}{7}b -a} \right){\text{ }}\left( { = (1 – \lambda )a + \frac{\lambda }{7}b} \right)\) M1A1

[2 marks]

b.i.

\(\overrightarrow {{\text{OD}}} = \frac{1}{2}\) a \( + \mu \left( { – \frac{1}{2}a + b} \right){\text{ }}\left( { = \left( {\frac{1}{2} – \frac{\mu }{2}} \right)a + \mu b} \right)\) M1A1

[2 marks]

b.ii.

equating coefficients: M1

\(\frac{\lambda }{7} = \mu ,{\text{ }}1 – \lambda = \frac{{1 – \mu }}{2}\) A1

solving simultaneously: M1

\(\lambda = \frac{7}{{13}},{\text{ }}\mu = \frac{1}{{13}}\) A1AG

[4 marks]

\(\overrightarrow {{\text{CD}}} = \frac{1}{{13}}\overrightarrow {{\text{CB}}} \)

\( = \frac{1}{{13}}\left( {b – \frac{1}{2}a} \right){\text{ }}\left( { = – \frac{1}{{26}}a + \frac{1}{{13}}b} \right)\) M1A1

[2 marks]

METHOD 1

\({\text{area }}\Delta {\text{ACD}} = \frac{1}{2}{\text{CD}} \times {\text{AC}} \times \sin {\rm{A\hat CB}}\) (M1)

\({\text{area }}\Delta {\text{ACB}} = \frac{1}{2}{\text{CB}} \times {\text{AC}} \times \sin {\rm{A\hat CB}}\) (M1)

\({\text{ratio }}\frac{{{\text{area }}\Delta {\text{ACD}}}}{{{\text{area }}\Delta {\text{ACB}}}} = \frac{{{\text{CD}}}}{{{\text{CB}}}} = \frac{1}{{13}}\) A1

\(k = \frac{{{\text{area }}\Delta {\text{OAB}}}}{{{\text{area }}\Delta {\text{CAD}}}} = \frac{{13}}{{{\text{area }}\Delta {\text{CAB}}}} \times {\text{area }}\Delta {\text{OAB}}\) (M1)

\( = 13 \times 2 = 26\) A1

METHOD 2

\({\text{area }}\Delta {\text{OAB}} = \frac{1}{2}\left| {a \times b} \right|\) A1

\( = \frac{1}{2}\left| {\frac{1}{2}a \times \left( { – \frac{1}{{26}}a + \frac{1}{{13}}b} \right)} \right|\)

\( = \frac{1}{2}\left| {\frac{1}{2}a \times \left( { – \frac{1}{{26}}a} \right) + \frac{1}{2}a \times \frac{1}{{13}}b} \right|\) (M1)

\( = \frac{1}{2} \times \frac{1}{2} \times \frac{1}{{13}}\left| {a \times b} \right|{\text{ }}\left( { = \frac{1}{{52}}\left| {a \times b} \right|} \right)\) A1

\({\text{area }}\Delta {\text{OAB}} = k({\text{area }}\Delta {\text{CAD}})\)

\(\frac{1}{2}\left| {a \times b} \right| = k\frac{1}{{52}}\left| {a \times b} \right|\)

\(k = 26\) A1

[5 marks]

Examiners report

[N/A]

a.i.

[N/A]

a.ii.

[N/A]

b.i.

[N/A]

b.ii.

[N/A]

Question

Find, in terms of a and b \(\overrightarrow {{\text{OF}}} \).

[1]

a.i.

Find, in terms of a and b \(\overrightarrow {{\text{AF}}} \).

[2]

a.ii.

Find an expression for \(\overrightarrow {{\text{OD}}} \) in terms of a, b and \(\lambda \);

[2]

b.i.

Find an expression for \(\overrightarrow {{\text{OD}}} \) in terms of a, b and \(\mu \).

[2]

b.ii.

Show that \(\mu = \frac{1}{{13}}\), and find the value of \(\lambda \).

[4]

Deduce an expression for \(\overrightarrow {{\text{CD}}} \) in terms of a and b only.

[2]

Given that area \(\Delta {\text{OAB}} = k({\text{area }}\Delta {\text{CAD}})\), find the value of \(k\).

[5]

Answer/Explanation

Markscheme

\(\overrightarrow {{\text{OF}}} = \frac{1}{7}\)b A1

[1 mark]

a.i.

\(\overrightarrow {{\text{AF}}} = \overrightarrow {{\text{OF}}} – \overrightarrow {{\text{OA}}} \) (M1)

\( = \frac{1}{7}\)b – a A1

[2 marks]

a.ii.

\(\overrightarrow {{\text{OD}}} = \) a \( + \lambda \left( {\frac{1}{7}b -a} \right){\text{ }}\left( { = (1 – \lambda )a + \frac{\lambda }{7}b} \right)\) M1A1

[2 marks]

b.i.

\(\overrightarrow {{\text{OD}}} = \frac{1}{2}\) a \( + \mu \left( { – \frac{1}{2}a + b} \right){\text{ }}\left( { = \left( {\frac{1}{2} – \frac{\mu }{2}} \right)a + \mu b} \right)\) M1A1

[2 marks]

b.ii.

equating coefficients: M1

\(\frac{\lambda }{7} = \mu ,{\text{ }}1 – \lambda = \frac{{1 – \mu }}{2}\) A1

solving simultaneously: M1

\(\lambda = \frac{7}{{13}},{\text{ }}\mu = \frac{1}{{13}}\) A1AG

[4 marks]

\(\overrightarrow {{\text{CD}}} = \frac{1}{{13}}\overrightarrow {{\text{CB}}} \)

\( = \frac{1}{{13}}\left( {b – \frac{1}{2}a} \right){\text{ }}\left( { = – \frac{1}{{26}}a + \frac{1}{{13}}b} \right)\) M1A1

[2 marks]

METHOD 1

\({\text{area }}\Delta {\text{ACD}} = \frac{1}{2}{\text{CD}} \times {\text{AC}} \times \sin {\rm{A\hat CB}}\) (M1)

\({\text{area }}\Delta {\text{ACB}} = \frac{1}{2}{\text{CB}} \times {\text{AC}} \times \sin {\rm{A\hat CB}}\) (M1)

\({\text{ratio }}\frac{{{\text{area }}\Delta {\text{ACD}}}}{{{\text{area }}\Delta {\text{ACB}}}} = \frac{{{\text{CD}}}}{{{\text{CB}}}} = \frac{1}{{13}}\) A1

\(k = \frac{{{\text{area }}\Delta {\text{OAB}}}}{{{\text{area }}\Delta {\text{CAD}}}} = \frac{{13}}{{{\text{area }}\Delta {\text{CAB}}}} \times {\text{area }}\Delta {\text{OAB}}\) (M1)

\( = 13 \times 2 = 26\) A1

METHOD 2

\({\text{area }}\Delta {\text{OAB}} = \frac{1}{2}\left| {a \times b} \right|\) A1

\( = \frac{1}{2}\left| {\frac{1}{2}a \times \left( { – \frac{1}{{26}}a + \frac{1}{{13}}b} \right)} \right|\)

\( = \frac{1}{2}\left| {\frac{1}{2}a \times \left( { – \frac{1}{{26}}a} \right) + \frac{1}{2}a \times \frac{1}{{13}}b} \right|\) (M1)

\( = \frac{1}{2} \times \frac{1}{2} \times \frac{1}{{13}}\left| {a \times b} \right|{\text{ }}\left( { = \frac{1}{{52}}\left| {a \times b} \right|} \right)\) A1

\({\text{area }}\Delta {\text{OAB}} = k({\text{area }}\Delta {\text{CAD}})\)

\(\frac{1}{2}\left| {a \times b} \right| = k\frac{1}{{52}}\left| {a \times b} \right|\)

\(k = 26\) A1

[5 marks]

Examiners report

[N/A]

a.i.

[N/A]

a.ii.

[N/A]

b.i.

[N/A]

b.ii.

[N/A]

Question

Find, in terms of a and b \(\overrightarrow {{\text{OF}}} \).

[1]

a.i.

Find, in terms of a and b \(\overrightarrow {{\text{AF}}} \).

[2]

a.ii.

Find an expression for \(\overrightarrow {{\text{OD}}} \) in terms of a, b and \(\lambda \);

[2]

b.i.

Find an expression for \(\overrightarrow {{\text{OD}}} \) in terms of a, b and \(\mu \).

[2]

b.ii.

Show that \(\mu = \frac{1}{{13}}\), and find the value of \(\lambda \).

[4]

Deduce an expression for \(\overrightarrow {{\text{CD}}} \) in terms of a and b only.

[2]

Given that area \(\Delta {\text{OAB}} = k({\text{area }}\Delta {\text{CAD}})\), find the value of \(k\).

[5]

Answer/Explanation

Markscheme

\(\overrightarrow {{\text{OF}}} = \frac{1}{7}\)b A1

[1 mark]

a.i.

\(\overrightarrow {{\text{AF}}} = \overrightarrow {{\text{OF}}} – \overrightarrow {{\text{OA}}} \) (M1)

\( = \frac{1}{7}\)b – a A1

[2 marks]

a.ii.

\(\overrightarrow {{\text{OD}}} = \) a \( + \lambda \left( {\frac{1}{7}b -a} \right){\text{ }}\left( { = (1 – \lambda )a + \frac{\lambda }{7}b} \right)\) M1A1

[2 marks]

b.i.

\(\overrightarrow {{\text{OD}}} = \frac{1}{2}\) a \( + \mu \left( { – \frac{1}{2}a + b} \right){\text{ }}\left( { = \left( {\frac{1}{2} – \frac{\mu }{2}} \right)a + \mu b} \right)\) M1A1

[2 marks]

b.ii.

equating coefficients: M1

\(\frac{\lambda }{7} = \mu ,{\text{ }}1 – \lambda = \frac{{1 – \mu }}{2}\) A1

solving simultaneously: M1

\(\lambda = \frac{7}{{13}},{\text{ }}\mu = \frac{1}{{13}}\) A1AG

[4 marks]

\(\overrightarrow {{\text{CD}}} = \frac{1}{{13}}\overrightarrow {{\text{CB}}} \)

\( = \frac{1}{{13}}\left( {b – \frac{1}{2}a} \right){\text{ }}\left( { = – \frac{1}{{26}}a + \frac{1}{{13}}b} \right)\) M1A1

[2 marks]

METHOD 1

\({\text{area }}\Delta {\text{ACD}} = \frac{1}{2}{\text{CD}} \times {\text{AC}} \times \sin {\rm{A\hat CB}}\) (M1)

\({\text{area }}\Delta {\text{ACB}} = \frac{1}{2}{\text{CB}} \times {\text{AC}} \times \sin {\rm{A\hat CB}}\) (M1)

\({\text{ratio }}\frac{{{\text{area }}\Delta {\text{ACD}}}}{{{\text{area }}\Delta {\text{ACB}}}} = \frac{{{\text{CD}}}}{{{\text{CB}}}} = \frac{1}{{13}}\) A1

\(k = \frac{{{\text{area }}\Delta {\text{OAB}}}}{{{\text{area }}\Delta {\text{CAD}}}} = \frac{{13}}{{{\text{area }}\Delta {\text{CAB}}}} \times {\text{area }}\Delta {\text{OAB}}\) (M1)

\( = 13 \times 2 = 26\) A1

METHOD 2

\({\text{area }}\Delta {\text{OAB}} = \frac{1}{2}\left| {a \times b} \right|\) A1

\( = \frac{1}{2}\left| {\frac{1}{2}a \times \left( { – \frac{1}{{26}}a + \frac{1}{{13}}b} \right)} \right|\)

\( = \frac{1}{2}\left| {\frac{1}{2}a \times \left( { – \frac{1}{{26}}a} \right) + \frac{1}{2}a \times \frac{1}{{13}}b} \right|\) (M1)

\( = \frac{1}{2} \times \frac{1}{2} \times \frac{1}{{13}}\left| {a \times b} \right|{\text{ }}\left( { = \frac{1}{{52}}\left| {a \times b} \right|} \right)\) A1

\({\text{area }}\Delta {\text{OAB}} = k({\text{area }}\Delta {\text{CAD}})\)

\(\frac{1}{2}\left| {a \times b} \right| = k\frac{1}{{52}}\left| {a \times b} \right|\)

\(k = 26\) A1

[5 marks]

Examiners report

[N/A]

a.i.

[N/A]

a.ii.

[N/A]

b.i.

[N/A]

b.ii.

[N/A]

Question

Find, in terms of a and b \(\overrightarrow {{\text{OF}}} \).

[1]

a.i.

Find, in terms of a and b \(\overrightarrow {{\text{AF}}} \).

[2]

a.ii.

Find an expression for \(\overrightarrow {{\text{OD}}} \) in terms of a, b and \(\lambda \);

[2]

b.i.

Find an expression for \(\overrightarrow {{\text{OD}}} \) in terms of a, b and \(\mu \).

[2]

b.ii.

Show that \(\mu = \frac{1}{{13}}\), and find the value of \(\lambda \).

[4]

Deduce an expression for \(\overrightarrow {{\text{CD}}} \) in terms of a and b only.

[2]

Given that area \(\Delta {\text{OAB}} = k({\text{area }}\Delta {\text{CAD}})\), find the value of \(k\).

[5]

Answer/Explanation

Markscheme

\(\overrightarrow {{\text{OF}}} = \frac{1}{7}\)b A1

[1 mark]

a.i.

\(\overrightarrow {{\text{AF}}} = \overrightarrow {{\text{OF}}} – \overrightarrow {{\text{OA}}} \) (M1)

\( = \frac{1}{7}\)b – a A1

[2 marks]

a.ii.

\(\overrightarrow {{\text{OD}}} = \) a \( + \lambda \left( {\frac{1}{7}b -a} \right){\text{ }}\left( { = (1 – \lambda )a + \frac{\lambda }{7}b} \right)\) M1A1

[2 marks]

b.i.

\(\overrightarrow {{\text{OD}}} = \frac{1}{2}\) a \( + \mu \left( { – \frac{1}{2}a + b} \right){\text{ }}\left( { = \left( {\frac{1}{2} – \frac{\mu }{2}} \right)a + \mu b} \right)\) M1A1

[2 marks]

b.ii.

equating coefficients: M1

\(\frac{\lambda }{7} = \mu ,{\text{ }}1 – \lambda = \frac{{1 – \mu }}{2}\) A1

solving simultaneously: M1

\(\lambda = \frac{7}{{13}},{\text{ }}\mu = \frac{1}{{13}}\) A1AG

[4 marks]

\(\overrightarrow {{\text{CD}}} = \frac{1}{{13}}\overrightarrow {{\text{CB}}} \)

\( = \frac{1}{{13}}\left( {b – \frac{1}{2}a} \right){\text{ }}\left( { = – \frac{1}{{26}}a + \frac{1}{{13}}b} \right)\) M1A1

[2 marks]

METHOD 1

\({\text{area }}\Delta {\text{ACD}} = \frac{1}{2}{\text{CD}} \times {\text{AC}} \times \sin {\rm{A\hat CB}}\) (M1)

\({\text{area }}\Delta {\text{ACB}} = \frac{1}{2}{\text{CB}} \times {\text{AC}} \times \sin {\rm{A\hat CB}}\) (M1)

\({\text{ratio }}\frac{{{\text{area }}\Delta {\text{ACD}}}}{{{\text{area }}\Delta {\text{ACB}}}} = \frac{{{\text{CD}}}}{{{\text{CB}}}} = \frac{1}{{13}}\) A1

\(k = \frac{{{\text{area }}\Delta {\text{OAB}}}}{{{\text{area }}\Delta {\text{CAD}}}} = \frac{{13}}{{{\text{area }}\Delta {\text{CAB}}}} \times {\text{area }}\Delta {\text{OAB}}\) (M1)

\( = 13 \times 2 = 26\) A1

METHOD 2

\({\text{area }}\Delta {\text{OAB}} = \frac{1}{2}\left| {a \times b} \right|\) A1

\( = \frac{1}{2}\left| {\frac{1}{2}a \times \left( { – \frac{1}{{26}}a + \frac{1}{{13}}b} \right)} \right|\)

\( = \frac{1}{2}\left| {\frac{1}{2}a \times \left( { – \frac{1}{{26}}a} \right) + \frac{1}{2}a \times \frac{1}{{13}}b} \right|\) (M1)

\( = \frac{1}{2} \times \frac{1}{2} \times \frac{1}{{13}}\left| {a \times b} \right|{\text{ }}\left( { = \frac{1}{{52}}\left| {a \times b} \right|} \right)\) A1

\({\text{area }}\Delta {\text{OAB}} = k({\text{area }}\Delta {\text{CAD}})\)

\(\frac{1}{2}\left| {a \times b} \right| = k\frac{1}{{52}}\left| {a \times b} \right|\)

\(k = 26\) A1

[5 marks]

Examiners report

[N/A]

a.i.

[N/A]

a.ii.

[N/A]

b.i.

[N/A]

b.ii.

[N/A]

Question

Find, in terms of a and b \(\overrightarrow {{\text{OF}}} \).

[1]

a.i.

Find, in terms of a and b \(\overrightarrow {{\text{AF}}} \).

[2]

a.ii.

Find an expression for \(\overrightarrow {{\text{OD}}} \) in terms of a, b and \(\lambda \);

[2]

b.i.

Find an expression for \(\overrightarrow {{\text{OD}}} \) in terms of a, b and \(\mu \).

[2]

b.ii.

Show that \(\mu = \frac{1}{{13}}\), and find the value of \(\lambda \).

[4]

Deduce an expression for \(\overrightarrow {{\text{CD}}} \) in terms of a and b only.

[2]

Given that area \(\Delta {\text{OAB}} = k({\text{area }}\Delta {\text{CAD}})\), find the value of \(k\).

[5]

Answer/Explanation

Markscheme

\(\overrightarrow {{\text{OF}}} = \frac{1}{7}\)b A1

[1 mark]

a.i.

\(\overrightarrow {{\text{AF}}} = \overrightarrow {{\text{OF}}} – \overrightarrow {{\text{OA}}} \) (M1)

\( = \frac{1}{7}\)b – a A1

[2 marks]

a.ii.

\(\overrightarrow {{\text{OD}}} = \) a \( + \lambda \left( {\frac{1}{7}b -a} \right){\text{ }}\left( { = (1 – \lambda )a + \frac{\lambda }{7}b} \right)\) M1A1

[2 marks]

b.i.

\(\overrightarrow {{\text{OD}}} = \frac{1}{2}\) a \( + \mu \left( { – \frac{1}{2}a + b} \right){\text{ }}\left( { = \left( {\frac{1}{2} – \frac{\mu }{2}} \right)a + \mu b} \right)\) M1A1

[2 marks]

b.ii.

equating coefficients: M1

\(\frac{\lambda }{7} = \mu ,{\text{ }}1 – \lambda = \frac{{1 – \mu }}{2}\) A1

solving simultaneously: M1

\(\lambda = \frac{7}{{13}},{\text{ }}\mu = \frac{1}{{13}}\) A1AG

[4 marks]

\(\overrightarrow {{\text{CD}}} = \frac{1}{{13}}\overrightarrow {{\text{CB}}} \)

\( = \frac{1}{{13}}\left( {b – \frac{1}{2}a} \right){\text{ }}\left( { = – \frac{1}{{26}}a + \frac{1}{{13}}b} \right)\) M1A1

[2 marks]

METHOD 1

\({\text{area }}\Delta {\text{ACD}} = \frac{1}{2}{\text{CD}} \times {\text{AC}} \times \sin {\rm{A\hat CB}}\) (M1)

\({\text{area }}\Delta {\text{ACB}} = \frac{1}{2}{\text{CB}} \times {\text{AC}} \times \sin {\rm{A\hat CB}}\) (M1)

\({\text{ratio }}\frac{{{\text{area }}\Delta {\text{ACD}}}}{{{\text{area }}\Delta {\text{ACB}}}} = \frac{{{\text{CD}}}}{{{\text{CB}}}} = \frac{1}{{13}}\) A1

\(k = \frac{{{\text{area }}\Delta {\text{OAB}}}}{{{\text{area }}\Delta {\text{CAD}}}} = \frac{{13}}{{{\text{area }}\Delta {\text{CAB}}}} \times {\text{area }}\Delta {\text{OAB}}\) (M1)

\( = 13 \times 2 = 26\) A1

METHOD 2

\({\text{area }}\Delta {\text{OAB}} = \frac{1}{2}\left| {a \times b} \right|\) A1

\( = \frac{1}{2}\left| {\frac{1}{2}a \times \left( { – \frac{1}{{26}}a + \frac{1}{{13}}b} \right)} \right|\)

\( = \frac{1}{2}\left| {\frac{1}{2}a \times \left( { – \frac{1}{{26}}a} \right) + \frac{1}{2}a \times \frac{1}{{13}}b} \right|\) (M1)

\( = \frac{1}{2} \times \frac{1}{2} \times \frac{1}{{13}}\left| {a \times b} \right|{\text{ }}\left( { = \frac{1}{{52}}\left| {a \times b} \right|} \right)\) A1

\({\text{area }}\Delta {\text{OAB}} = k({\text{area }}\Delta {\text{CAD}})\)

\(\frac{1}{2}\left| {a \times b} \right| = k\frac{1}{{52}}\left| {a \times b} \right|\)

\(k = 26\) A1

[5 marks]

Examiners report

[N/A]

a.i.

[N/A]

a.ii.

[N/A]

b.i.

[N/A]

b.ii.

[N/A]

Question

Find, in terms of a and b \(\overrightarrow {{\text{OF}}} \).

[1]

a.i.

Find, in terms of a and b \(\overrightarrow {{\text{AF}}} \).

[2]

a.ii.

Find an expression for \(\overrightarrow {{\text{OD}}} \) in terms of a, b and \(\lambda \);

[2]

b.i.

Find an expression for \(\overrightarrow {{\text{OD}}} \) in terms of a, b and \(\mu \).

[2]

b.ii.

Show that \(\mu = \frac{1}{{13}}\), and find the value of \(\lambda \).

[4]

Deduce an expression for \(\overrightarrow {{\text{CD}}} \) in terms of a and b only.

[2]

Given that area \(\Delta {\text{OAB}} = k({\text{area }}\Delta {\text{CAD}})\), find the value of \(k\).

[5]

Answer/Explanation

Markscheme

\(\overrightarrow {{\text{OF}}} = \frac{1}{7}\)b A1

[1 mark]

a.i.

\(\overrightarrow {{\text{AF}}} = \overrightarrow {{\text{OF}}} – \overrightarrow {{\text{OA}}} \) (M1)

\( = \frac{1}{7}\)b – a A1

[2 marks]

a.ii.

\(\overrightarrow {{\text{OD}}} = \) a \( + \lambda \left( {\frac{1}{7}b -a} \right){\text{ }}\left( { = (1 – \lambda )a + \frac{\lambda }{7}b} \right)\) M1A1

[2 marks]

b.i.

\(\overrightarrow {{\text{OD}}} = \frac{1}{2}\) a \( + \mu \left( { – \frac{1}{2}a + b} \right){\text{ }}\left( { = \left( {\frac{1}{2} – \frac{\mu }{2}} \right)a + \mu b} \right)\) M1A1

[2 marks]

b.ii.

equating coefficients: M1

\(\frac{\lambda }{7} = \mu ,{\text{ }}1 – \lambda = \frac{{1 – \mu }}{2}\) A1

solving simultaneously: M1

\(\lambda = \frac{7}{{13}},{\text{ }}\mu = \frac{1}{{13}}\) A1AG

[4 marks]

\(\overrightarrow {{\text{CD}}} = \frac{1}{{13}}\overrightarrow {{\text{CB}}} \)

\( = \frac{1}{{13}}\left( {b – \frac{1}{2}a} \right){\text{ }}\left( { = – \frac{1}{{26}}a + \frac{1}{{13}}b} \right)\) M1A1

[2 marks]

METHOD 1

\({\text{area }}\Delta {\text{ACD}} = \frac{1}{2}{\text{CD}} \times {\text{AC}} \times \sin {\rm{A\hat CB}}\) (M1)

\({\text{area }}\Delta {\text{ACB}} = \frac{1}{2}{\text{CB}} \times {\text{AC}} \times \sin {\rm{A\hat CB}}\) (M1)

\({\text{ratio }}\frac{{{\text{area }}\Delta {\text{ACD}}}}{{{\text{area }}\Delta {\text{ACB}}}} = \frac{{{\text{CD}}}}{{{\text{CB}}}} = \frac{1}{{13}}\) A1

\(k = \frac{{{\text{area }}\Delta {\text{OAB}}}}{{{\text{area }}\Delta {\text{CAD}}}} = \frac{{13}}{{{\text{area }}\Delta {\text{CAB}}}} \times {\text{area }}\Delta {\text{OAB}}\) (M1)

\( = 13 \times 2 = 26\) A1

METHOD 2

\({\text{area }}\Delta {\text{OAB}} = \frac{1}{2}\left| {a \times b} \right|\) A1

\( = \frac{1}{2}\left| {\frac{1}{2}a \times \left( { – \frac{1}{{26}}a + \frac{1}{{13}}b} \right)} \right|\)

\( = \frac{1}{2}\left| {\frac{1}{2}a \times \left( { – \frac{1}{{26}}a} \right) + \frac{1}{2}a \times \frac{1}{{13}}b} \right|\) (M1)

\( = \frac{1}{2} \times \frac{1}{2} \times \frac{1}{{13}}\left| {a \times b} \right|{\text{ }}\left( { = \frac{1}{{52}}\left| {a \times b} \right|} \right)\) A1

\({\text{area }}\Delta {\text{OAB}} = k({\text{area }}\Delta {\text{CAD}})\)

\(\frac{1}{2}\left| {a \times b} \right| = k\frac{1}{{52}}\left| {a \times b} \right|\)

\(k = 26\) A1

[5 marks]

Examiners report

[N/A]

a.i.

[N/A]

a.ii.

[N/A]

b.i.

[N/A]

b.ii.

[N/A]

Question

The points A, B, C and D have position vectors a, b, c and d, relative to the origin O.

It is given that \(\mathop {{\text{AB}}}\limits^ \to = \mathop {{\text{DC}}}\limits^ \to \).

The position vectors \(\mathop {{\text{OA}}}\limits^ \to \), \(\mathop {{\text{OB}}}\limits^ \to \), \(\mathop {{\text{OC}}}\limits^ \to \) and \(\mathop {{\text{OD}}}\limits^ \to \) are given by

a = i + 2j − 3k

b = 3i − j + pk

c = qi + j + 2k

d = −i + rj − 2k

where p , q and r are constants.

The point where the diagonals of ABCD intersect is denoted by M.

The plane \(\Pi \) cuts the x, y and z axes at X , Y and Z respectively.

Explain why ABCD is a parallelogram.

[1]

a.i.

Using vector algebra, show that \(\mathop {{\text{AD}}}\limits^ \to = \mathop {{\text{BC}}}\limits^ \to \).

[3]

a.ii.

Show that p = 1, q = 1 and r = 4.

[5]

Find the area of the parallelogram ABCD.

[4]

Find the vector equation of the straight line passing through M and normal to the plane \(\Pi \) containing ABCD.

[4]

Find the Cartesian equation of \(\Pi \).

[3]

Find the coordinates of X, Y and Z.

[2]

f.i.

Find YZ.

[2]

f.ii.

Answer/Explanation

Markscheme

a pair of opposite sides have equal length and are parallel R1

hence ABCD is a parallelogram AG

[1 mark]

a.i.

attempt to rewrite the given information in vector form M1

b − a = c − d A1

rearranging d − a = c − b M1

hence \(\mathop {{\text{AD}}}\limits^ \to = \mathop {{\text{BC}}}\limits^ \to \) AG

Note: Candidates may correctly answer part i) by answering part ii) correctly and then deducing there
are two pairs of parallel sides.

[3 marks]

a.ii.

EITHER

use of \(\mathop {{\text{AB}}}\limits^ \to = \mathop {{\text{DC}}}\limits^ \to \) (M1)

\(\left( \begin{gathered}
2 \hfill \\
– 3 \hfill \\
p + 3 \hfill \\
\end{gathered} \right) = \left( \begin{gathered}
q + 1 \hfill \\
1 – r \hfill \\
4 \hfill \\
\end{gathered} \right)\) A1A1

use of \(\mathop {{\text{AD}}}\limits^ \to = \mathop {{\text{BC}}}\limits^ \to \) (M1)

\(\left( \begin{gathered}
– 2 \hfill \\
r – 2 \hfill \\
1 \hfill \\
\end{gathered} \right) = \left( \begin{gathered}
q – 3 \hfill \\
2 \hfill \\
2 – p \hfill \\
\end{gathered} \right)\) A1A1

THEN

attempt to compare coefficients of i, j, and k in their equation or statement to that effect M1

clear demonstration that the given values satisfy their equation A1
p = 1, q = 1, r = 4 AG

[5 marks]

attempt at computing \(\mathop {{\text{AB}}}\limits^ \to \, \times \mathop {{\text{AD}}}\limits^ \to \) (or equivalent) M1

\(\left( \begin{gathered}
– 11 \hfill \\
– 10 \hfill \\
– 2 \hfill \\
\end{gathered} \right)\) A1

area \( = \left| {\mathop {{\text{AB}}}\limits^ \to \, \times \mathop {{\text{AD}}}\limits^ \to } \right|\left( { = \sqrt {225} } \right)\) (M1)

= 15 A1

[4 marks]

valid attempt to find \(\mathop {{\text{OM}}}\limits^ \to = \left( {\frac{1}{2}\left( {a + c} \right)} \right)\) (M1)

\(\left( \begin{gathered}
1 \hfill \\
\frac{3}{2} \hfill \\
– \frac{1}{2} \hfill \\
\end{gathered} \right)\) A1

the equation is

r = \(\left( \begin{gathered}
1 \hfill \\
\frac{3}{2} \hfill \\
– \frac{1}{2} \hfill \\
\end{gathered} \right) + t\left( \begin{gathered}
11 \hfill \\
10 \hfill \\
2 \hfill \\
\end{gathered} \right)\) or equivalent M1A1

Note: Award maximum M1A0 if ‘r = …’ (or equivalent) is not seen.

[4 marks]

attempt to obtain the equation of the plane in the form ax + by + cz = d M1

11x + 10y + 2z = 25 A1A1

Note: A1 for right hand side, A1 for left hand side.

[3 marks]

putting two coordinates equal to zero (M1)

\({\text{X}}\left( {\frac{{25}}{{11}},\,0,\,0} \right),\,\,{\text{Y}}\left( {0,\,\frac{5}{2},\,0} \right),\,\,{\text{Z}}\left( {0,\,0,\,\frac{{25}}{2}} \right)\) A1

[2 marks]

f.i.

\({\text{YZ}} = \sqrt {{{\left( {\frac{5}{2}} \right)}^2} + {{\left( {\frac{{25}}{2}} \right)}^2}} \) M1

\( = \sqrt {\frac{{325}}{2}} \left( { = \frac{{5\sqrt {104} }}{4} = \frac{{5\sqrt {26} }}{2}} \right)\) A1

[4 marks]

f.ii.

Examiners report

[N/A]

a.i.

[N/A]

a.ii.

[N/A]

f.i.

[N/A]

f.ii.

Question

The points A, B, C and D have position vectors a, b, c and d, relative to the origin O.

It is given that \(\mathop {{\text{AB}}}\limits^ \to = \mathop {{\text{DC}}}\limits^ \to \).

The position vectors \(\mathop {{\text{OA}}}\limits^ \to \), \(\mathop {{\text{OB}}}\limits^ \to \), \(\mathop {{\text{OC}}}\limits^ \to \) and \(\mathop {{\text{OD}}}\limits^ \to \) are given by

a = i + 2j − 3k

b = 3i − j + pk

c = qi + j + 2k

d = −i + rj − 2k

where p , q and r are constants.

The point where the diagonals of ABCD intersect is denoted by M.

The plane \(\Pi \) cuts the x, y and z axes at X , Y and Z respectively.

Explain why ABCD is a parallelogram.

[1]

a.i.

Using vector algebra, show that \(\mathop {{\text{AD}}}\limits^ \to = \mathop {{\text{BC}}}\limits^ \to \).

[3]

a.ii.

Show that p = 1, q = 1 and r = 4.

[5]

Find the area of the parallelogram ABCD.

[4]

Find the vector equation of the straight line passing through M and normal to the plane \(\Pi \) containing ABCD.

[4]

Find the Cartesian equation of \(\Pi \).

[3]

Find the coordinates of X, Y and Z.

[2]

f.i.

Find YZ.

[2]

f.ii.

Answer/Explanation

Markscheme

a pair of opposite sides have equal length and are parallel R1

hence ABCD is a parallelogram AG

[1 mark]

a.i.

attempt to rewrite the given information in vector form M1

b − a = c − d A1

rearranging d − a = c − b M1

hence \(\mathop {{\text{AD}}}\limits^ \to = \mathop {{\text{BC}}}\limits^ \to \) AG

Note: Candidates may correctly answer part i) by answering part ii) correctly and then deducing there
are two pairs of parallel sides.

[3 marks]

a.ii.

EITHER

use of \(\mathop {{\text{AB}}}\limits^ \to = \mathop {{\text{DC}}}\limits^ \to \) (M1)

\(\left( \begin{gathered}
2 \hfill \\
– 3 \hfill \\
p + 3 \hfill \\
\end{gathered} \right) = \left( \begin{gathered}
q + 1 \hfill \\
1 – r \hfill \\
4 \hfill \\
\end{gathered} \right)\) A1A1

use of \(\mathop {{\text{AD}}}\limits^ \to = \mathop {{\text{BC}}}\limits^ \to \) (M1)

THEN

attempt to compare coefficients of i, j, and k in their equation or statement to that effect M1

clear demonstration that the given values satisfy their equation A1
p = 1, q = 1, r = 4 AG

[5 marks]

attempt at computing \(\mathop {{\text{AB}}}\limits^ \to \, \times \mathop {{\text{AD}}}\limits^ \to \) (or equivalent) M1

\(\left( \begin{gathered}
– 11 \hfill \\
– 10 \hfill \\
– 2 \hfill \\
\end{gathered} \right)\) A1

area \( = \left| {\mathop {{\text{AB}}}\limits^ \to \, \times \mathop {{\text{AD}}}\limits^ \to } \right|\left( { = \sqrt {225} } \right)\) (M1)

= 15 A1

[4 marks]

valid attempt to find \(\mathop {{\text{OM}}}\limits^ \to = \left( {\frac{1}{2}\left( {a + c} \right)} \right)\) (M1)

\(\left( \begin{gathered}
1 \hfill \\
\frac{3}{2} \hfill \\
– \frac{1}{2} \hfill \\
\end{gathered} \right)\) A1

the equation is

Note: Award maximum M1A0 if ‘r = …’ (or equivalent) is not seen.

[4 marks]

attempt to obtain the equation of the plane in the form ax + by + cz = d M1

11x + 10y + 2z = 25 A1A1

Note: A1 for right hand side, A1 for left hand side.

[3 marks]

putting two coordinates equal to zero (M1)

\({\text{X}}\left( {\frac{{25}}{{11}},\,0,\,0} \right),\,\,{\text{Y}}\left( {0,\,\frac{5}{2},\,0} \right),\,\,{\text{Z}}\left( {0,\,0,\,\frac{{25}}{2}} \right)\) A1

[2 marks]

f.i.

\({\text{YZ}} = \sqrt {{{\left( {\frac{5}{2}} \right)}^2} + {{\left( {\frac{{25}}{2}} \right)}^2}} \) M1

\( = \sqrt {\frac{{325}}{2}} \left( { = \frac{{5\sqrt {104} }}{4} = \frac{{5\sqrt {26} }}{2}} \right)\) A1

[4 marks]

f.ii.

Examiners report

[N/A]

a.i.

[N/A]

a.ii.

[N/A]

f.i.

[N/A]

f.ii.

Question

The points A, B, C and D have position vectors a, b, c and d, relative to the origin O.

It is given that \(\mathop {{\text{AB}}}\limits^ \to = \mathop {{\text{DC}}}\limits^ \to \).

The position vectors \(\mathop {{\text{OA}}}\limits^ \to \), \(\mathop {{\text{OB}}}\limits^ \to \), \(\mathop {{\text{OC}}}\limits^ \to \) and \(\mathop {{\text{OD}}}\limits^ \to \) are given by

a = i + 2j − 3k

b = 3i − j + pk

c = qi + j + 2k

d = −i + rj − 2k

where p , q and r are constants.

The point where the diagonals of ABCD intersect is denoted by M.

The plane \(\Pi \) cuts the x, y and z axes at X , Y and Z respectively.

Explain why ABCD is a parallelogram.

[1]

a.i.

Using vector algebra, show that \(\mathop {{\text{AD}}}\limits^ \to = \mathop {{\text{BC}}}\limits^ \to \).

[3]

a.ii.

Show that p = 1, q = 1 and r = 4.

[5]

Find the area of the parallelogram ABCD.

[4]

Find the vector equation of the straight line passing through M and normal to the plane \(\Pi \) containing ABCD.

[4]

Find the Cartesian equation of \(\Pi \).

[3]

Find the coordinates of X, Y and Z.

[2]

f.i.

Find YZ.

[2]

f.ii.

Answer/Explanation

Markscheme

a pair of opposite sides have equal length and are parallel R1

hence ABCD is a parallelogram AG

[1 mark]

a.i.

attempt to rewrite the given information in vector form M1

b − a = c − d A1

rearranging d − a = c − b M1

hence \(\mathop {{\text{AD}}}\limits^ \to = \mathop {{\text{BC}}}\limits^ \to \) AG

Note: Candidates may correctly answer part i) by answering part ii) correctly and then deducing there
are two pairs of parallel sides.

[3 marks]

a.ii.

EITHER

use of \(\mathop {{\text{AB}}}\limits^ \to = \mathop {{\text{DC}}}\limits^ \to \) (M1)

\(\left( \begin{gathered}
2 \hfill \\
– 3 \hfill \\
p + 3 \hfill \\
\end{gathered} \right) = \left( \begin{gathered}
q + 1 \hfill \\
1 – r \hfill \\
4 \hfill \\
\end{gathered} \right)\) A1A1

use of \(\mathop {{\text{AD}}}\limits^ \to = \mathop {{\text{BC}}}\limits^ \to \) (M1)

THEN

attempt to compare coefficients of i, j, and k in their equation or statement to that effect M1

clear demonstration that the given values satisfy their equation A1
p = 1, q = 1, r = 4 AG

[5 marks]

attempt at computing \(\mathop {{\text{AB}}}\limits^ \to \, \times \mathop {{\text{AD}}}\limits^ \to \) (or equivalent) M1

\(\left( \begin{gathered}
– 11 \hfill \\
– 10 \hfill \\
– 2 \hfill \\
\end{gathered} \right)\) A1

area \( = \left| {\mathop {{\text{AB}}}\limits^ \to \, \times \mathop {{\text{AD}}}\limits^ \to } \right|\left( { = \sqrt {225} } \right)\) (M1)

= 15 A1

[4 marks]

valid attempt to find \(\mathop {{\text{OM}}}\limits^ \to = \left( {\frac{1}{2}\left( {a + c} \right)} \right)\) (M1)

\(\left( \begin{gathered}
1 \hfill \\
\frac{3}{2} \hfill \\
– \frac{1}{2} \hfill \\
\end{gathered} \right)\) A1

the equation is

Note: Award maximum M1A0 if ‘r = …’ (or equivalent) is not seen.

[4 marks]

attempt to obtain the equation of the plane in the form ax + by + cz = d M1

11x + 10y + 2z = 25 A1A1

Note: A1 for right hand side, A1 for left hand side.

[3 marks]

putting two coordinates equal to zero (M1)

\({\text{X}}\left( {\frac{{25}}{{11}},\,0,\,0} \right),\,\,{\text{Y}}\left( {0,\,\frac{5}{2},\,0} \right),\,\,{\text{Z}}\left( {0,\,0,\,\frac{{25}}{2}} \right)\) A1

[2 marks]

f.i.

\({\text{YZ}} = \sqrt {{{\left( {\frac{5}{2}} \right)}^2} + {{\left( {\frac{{25}}{2}} \right)}^2}} \) M1

\( = \sqrt {\frac{{325}}{2}} \left( { = \frac{{5\sqrt {104} }}{4} = \frac{{5\sqrt {26} }}{2}} \right)\) A1

[4 marks]

f.ii.

Examiners report

[N/A]

a.i.

[N/A]

a.ii.

[N/A]

f.i.

[N/A]

f.ii.

Question

The points A, B, C and D have position vectors a, b, c and d, relative to the origin O.

It is given that \(\mathop {{\text{AB}}}\limits^ \to = \mathop {{\text{DC}}}\limits^ \to \).

The position vectors \(\mathop {{\text{OA}}}\limits^ \to \), \(\mathop {{\text{OB}}}\limits^ \to \), \(\mathop {{\text{OC}}}\limits^ \to \) and \(\mathop {{\text{OD}}}\limits^ \to \) are given by

a = i + 2j − 3k

b = 3i − j + pk

c = qi + j + 2k

d = −i + rj − 2k

where p , q and r are constants.

The point where the diagonals of ABCD intersect is denoted by M.

The plane \(\Pi \) cuts the x, y and z axes at X , Y and Z respectively.

Explain why ABCD is a parallelogram.

[1]

a.i.

Using vector algebra, show that \(\mathop {{\text{AD}}}\limits^ \to = \mathop {{\text{BC}}}\limits^ \to \).

[3]

a.ii.

Show that p = 1, q = 1 and r = 4.

[5]

Find the area of the parallelogram ABCD.

[4]

Find the vector equation of the straight line passing through M and normal to the plane \(\Pi \) containing ABCD.

[4]

Find the Cartesian equation of \(\Pi \).

[3]

Find the coordinates of X, Y and Z.

[2]

f.i.

Find YZ.

[2]

f.ii.

Answer/Explanation

Markscheme

a pair of opposite sides have equal length and are parallel R1

hence ABCD is a parallelogram AG

[1 mark]

a.i.

attempt to rewrite the given information in vector form M1

b − a = c − d A1

rearranging d − a = c − b M1

hence \(\mathop {{\text{AD}}}\limits^ \to = \mathop {{\text{BC}}}\limits^ \to \) AG

Note: Candidates may correctly answer part i) by answering part ii) correctly and then deducing there
are two pairs of parallel sides.

[3 marks]

a.ii.

EITHER

use of \(\mathop {{\text{AB}}}\limits^ \to = \mathop {{\text{DC}}}\limits^ \to \) (M1)

\(\left( \begin{gathered}
2 \hfill \\
– 3 \hfill \\
p + 3 \hfill \\
\end{gathered} \right) = \left( \begin{gathered}
q + 1 \hfill \\
1 – r \hfill \\
4 \hfill \\
\end{gathered} \right)\) A1A1

use of \(\mathop {{\text{AD}}}\limits^ \to = \mathop {{\text{BC}}}\limits^ \to \) (M1)

THEN

attempt to compare coefficients of i, j, and k in their equation or statement to that effect M1

clear demonstration that the given values satisfy their equation A1
p = 1, q = 1, r = 4 AG

[5 marks]

attempt at computing \(\mathop {{\text{AB}}}\limits^ \to \, \times \mathop {{\text{AD}}}\limits^ \to \) (or equivalent) M1

\(\left( \begin{gathered}
– 11 \hfill \\
– 10 \hfill \\
– 2 \hfill \\
\end{gathered} \right)\) A1

area \( = \left| {\mathop {{\text{AB}}}\limits^ \to \, \times \mathop {{\text{AD}}}\limits^ \to } \right|\left( { = \sqrt {225} } \right)\) (M1)

= 15 A1

[4 marks]

valid attempt to find \(\mathop {{\text{OM}}}\limits^ \to = \left( {\frac{1}{2}\left( {a + c} \right)} \right)\) (M1)

\(\left( \begin{gathered}
1 \hfill \\
\frac{3}{2} \hfill \\
– \frac{1}{2} \hfill \\
\end{gathered} \right)\) A1

the equation is

Note: Award maximum M1A0 if ‘r = …’ (or equivalent) is not seen.

[4 marks]

attempt to obtain the equation of the plane in the form ax + by + cz = d M1

11x + 10y + 2z = 25 A1A1

Note: A1 for right hand side, A1 for left hand side.

[3 marks]

putting two coordinates equal to zero (M1)

\({\text{X}}\left( {\frac{{25}}{{11}},\,0,\,0} \right),\,\,{\text{Y}}\left( {0,\,\frac{5}{2},\,0} \right),\,\,{\text{Z}}\left( {0,\,0,\,\frac{{25}}{2}} \right)\) A1

[2 marks]

f.i.

\({\text{YZ}} = \sqrt {{{\left( {\frac{5}{2}} \right)}^2} + {{\left( {\frac{{25}}{2}} \right)}^2}} \) M1

\( = \sqrt {\frac{{325}}{2}} \left( { = \frac{{5\sqrt {104} }}{4} = \frac{{5\sqrt {26} }}{2}} \right)\) A1

[4 marks]

f.ii.

Examiners report

[N/A]

a.i.

[N/A]

a.ii.

[N/A]

f.i.

[N/A]

f.ii.

Question

Markscheme

Examiners report

Question

Markscheme

Examiners report

Question

Markscheme

Examiners report

Question

Markscheme

Examiners report

Question

Markscheme

Examiners report

Question

Markscheme

Examiners report

Question

Markscheme

Examiners report

Question

Markscheme

Examiners report

Question

Markscheme

Examiners report

Question

Markscheme

Examiners report

Question

Markscheme

Examiners report

Question

Markscheme

Examiners report

Question

Markscheme

Examiners report

Question

Markscheme

Examiners report

Question

Markscheme

Examiners report

Question

Markscheme

Examiners report

Question

Markscheme

Examiners report

Question

Markscheme

Examiners report

Question

Markscheme

Examiners report

Resources

Members

Company