Download IITian Academy App for accessing Online Mock Tests and More..
Question
The strength of beams compared against the moisture content of the beam is indicated in the following table. You should assume that strength and moisture content are each normally distributed.
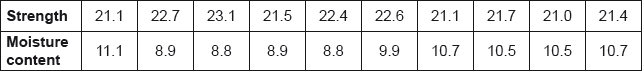
a.Determine the product moment correlation coefficient for these data.[2]
b.Perform a two-tailed test, at the \(5\% \) level of significance, of the hypothesis that strength is independent of moisture content.[5]
c.If the moisture content of a beam is found to be \(9.5\), use the appropriate regression line to estimate the strength of the beam.[4]
Answer/Explanation
Markscheme
\(r = – 0.762\) (M1)A1
Note: Accept answers that round to \( – 0.76\).
[2 marks]
\({H_0}:\) Moisture content and strength are independent or \(\rho = 0\)
\({H_1}:\) Moisture content and strength are not independent or \(\rho \ne 0\) A1
EITHER
test statistic is \(-3.33\) A1
critical value is \(( \pm ){\text{ }}2.306\) A1
since \( – 3.33 < – 2.306\) or \(3.33 > 2.306\), R1
reject \({H_0}\;\;\;\)(or equivalent) A1
OR
\(p\)-value is \(0.0104\) A2
as \(0.0104 < 0.05\), R1
reject \({H_0}\;\;\;\)(or equivalent) A1
Note: The R1 and A1 can be awarded as follow through from their test statistic or \(p\)-value.
[5 marks]
\(x = {\text{strength}}\)
\(y = {\text{moisture content}}\)
\(x = – 0.629y + 28.1\) (M1)(A1)
if \(y = 9.5\) so \(x = 22.1\) (M1)A1
Note: Only accept answers that round to \(22.1\).
Note: Award M1A1M0A0 for the other regression line \(y = 30.1 – 0.924x\).
[4 marks]
Total [11 marks]
Examiners report
[N/A]
[N/A]
[N/A]